ON THE ART AND SCIENCE OF ACOUSTIC INSTRUMENTS
© 2000–2025 Cris Forster
Two definitions at Just Intonation provide background information
for the following discussions.
For descriptions of the arithmetic and harmonic divisions of musical intervals
that determine the ‘minor’ and ‘major’ tonalities in Western music, see
Ibn Sina, Stifel, and Zarlino.
CHAPTER 3: FLEXIBLE STRINGS
Part V: Musical, Mathematical, and Linguistic Origins of Length Ratios
Section 3.16
We may use the preceding example, which describes the tuning process of two unfamiliar frequency ratios, 7/5 and 7/2, to analyze the musical, mathematical, and linguistic origins of length ratios. Although aural experiences of sound depend on frequencies, our anatomy does not enable us to quantify rates of vibration from oscillating strings. As such, the frequencies produced while playing musical intervals have always and will always remain incomprehensible quantities. Therefore, if we spontaneously tune two canons strings to an unfamiliar musical interval, we cannot identify the new interval by counting cycles per second and constructing a frequency ratio. To comprehend such an experience, we must measure the corresponding string lengths, and through the construction of a length ratio, identify the unfamiliar interval. Conversely, to intentionally tune two strings to a new musical interval, we cannot locate the required position of a canon bridge by simply listening to frequencies. Instead, we must divide the strings according to a length ratio that determines the frequency ratio we have not yet experienced. In both of these cases, our intellectual ability to comprehend aurally perceived frequency ratios depends on our mathematical ability to measure and compare string lengths.
We may summarize the preceding discussion by referring to Equation 3.3, which states that frequency is a function of wavelength. Before we analyze this statement further, let us first examine modern mathematical notation with respect to the term “function.” Typically, a function constitutes a cause-and-effect relation between two variables x and y, where the x-number, chosen randomly or at will, is called the independent variable, and the y-number, determined only after the x-number has been selected, is called the dependent variable. For example, if we state that the area of circle (y) equals π (3.1416) times the radius (x) squared, then the expression y = πx2 defines y as a function of x, or the area as a function of the radius. To specify this functional relation, mathematicians replace y with x), which reads, “ƒ of x,” or stated in full, “the value of the function ƒ at x.” Therefore, the previous equation in functional notation states ƒ(x) = πx2. This notation informs us that in the functional relation between variables x and y, values of x determine values of y. Now, substitute the radius x = 2 into these two equations, and calculate the area y:
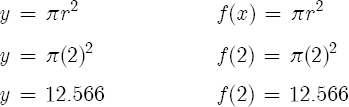
In the left column, a sequence of equations simply terminates in a solution for the area y, whereas in the right column, a sequence of equations in functional notation terminates not only in a solution, but indicates the value of radius x that determines area y.
If we solve this equation for x, the expression x = √(y/π) defines x as a function of y, or the radius as a function of the area. Therefore, in functional notation: ƒ(y) = √(y/π). Substitute the area y = 4 into these two equations, and calculate the radius x:
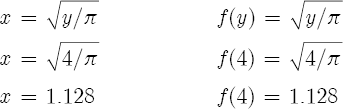
Now, recall Equation 3.3,
![]()
and express F (frequency) as a function of λ (wavelength):
![]()
Next, solve Equation 3.3 for λ,
![]()
and express λ as a function of F:
![]()
Equation 3.36 expresses a functional relation that is algebraically and scientifically correct: as frequencies increase, wavelengths decrease; vice versa. However, since we cannot count rates of vibration from oscillating strings, we cannot substitute values for the independent variable F into this equation. That is, our aural experience of an unfamiliar interval does not enable us to quantify a frequency ratio, which we could then invert to identify the corresponding length ratio.
In contrast, Equation 3.35 essentially describes the musical and mathematical process by which musicians create ascending sequences of tones that result in tuned scales. By shortening the lengths of strings in precise increments, as wavelengths decrease, frequencies increase. This tuning process requires two steps. (1) Our anatomy enables us to measure, quantify, and comprehend all changes in string lengths. (2) The inverse proportionality between length ratios and frequency ratios — l.r. x/n → f.r. n/x — permits us to interpret these length ratios as frequency ratios, which we then identify as musical intervals. This second step is crucial simply because we do not hear wavelengths or string lengths. However, note carefully that we also do not hear f.r. 3/2 as an auditory phenomenon where one frequency vibrates 1.5 times faster than another frequency. Instead, to give meaning to such an aural experience, we define f.r. 3/2 as a musical interval called the “fifth.” Although for known musical intervals, we may notate the inverse proportionality between frequency ratios and length ratios — f.r. n/x → l.r. x/n — music as an exploratory science and as a meaningful art principally depends on the knowledge acquired through comprehensible string length measurements and on the construction of length ratios.
Furthermore, in the context of the harmonic series, recall that the superposition of traveling waves causes nodes in strings. These internal obstructions cause decreases in loop lengths, which in turn cause decreases in wavelengths: as wavelengths decrease, mode frequencies increase. From this perspective, Equation 3.35 expresses the natural process by which a string, through a harmonic progression of length ratios 1, 1/2, 1/3, 1/4, 1/5, 1/6, . . . , shortens itself![1] We conclude, therefore, that in the scientific relationship between vibrating strings and the internal processes of subdivision, mode frequencies are a function of mode wavelengths; and in the historical relationship between musicians and the mathematics of canon string divisions, frequency ratios are a function of length ratios. Stated differently, for a constant transverse wave speed, length ratios constitute the primary factor in identifying, determining, and controlling frequency ratios.
Section 3.17
The Greeks primarily used verbal terms to describe mathematical ratios. Furthermore, due to linguistic complications, the Greeks never employed fractions such as 1/2, 2/3, 3/4, 8/9, etc., to identify musical intervals. Instead, the ratios of Greek music always express quotients greater than one. For example, examine Figure 3.21 and observe that the term for the ratio of the “octave” (or diapason) is diplasios. Since the prefix di means two, and plasma means something formed, diplasios means twofold, or double, as in 1 + 1 = 1/1 x 2 = 2/1. Therefore, the “octave” is a musical interval produced by a string that is twice as long as another string; hence, l.r. 2/1. Similarly, the term for the ratio of the “fifth” (or diapente) is hemiolios. Since the prefix hemi means half, and olos means whole, hemiolios means half and whole: 1/2 + 1 = 1/2 + 2/2 = 3/2. Therefore, the “fifth” is a musical interval produced by a string that is by a half longer than another string; hence, l.r. 3/2. The Greek term for the ratio of the “fourth” (or diatessaron) is epitritos. Since, in a mathematical context, the prefix epi describes the operation of addition,[2] and tritos means third, the term epitritos denotes one-third in addition, and connotes one and one-third: 1 + 1/3 = 3/3 + 1/3 = 4/3. Therefore, the “fourth” is a musical interval produced by a string that is by a third longer than another string; hence, l.r. 4/3. Similarly, the term for the ratio of the “whole tone” (or tonon) is epogdoos (or epiogdoos) which connotes one and one-eighth: 1 + 1/8 = 8/8 + 1/8 = 9/8. Therefore, the “whole tone” is a musical interval produced by a string that is by an eighth longer than another string; hence, l.r. 9/8.

In contrast, to notate common ratios whose quotients are less than one, the Greeks inverted these ratios by attaching a prefix hyp or hyph, from hypo, which means under, as in the reciprocal of a number, or 1/x. Therefore, hypodiplasios means half, or 1 ÷ 2 = 1/2; hyphhemiolios means two-thirds, or 1 ÷ (1 + 1/2) = 2/3; hypepitritos means three-fourths, or 1 ÷ (1 + 1/3) = 3/4; and hypepogdoos means eight-ninths, or 1 ÷ (1 + 1/8) = 8/9.[3] These kinds of ratios occurred very rarely in the context of canon string divisions, and were never used to identify musical intervals.
In his translation of Euclid’s Division of the Canon, André Barbera observes
If the long version of the Division of the Canon originated in the fourth century B.C., it contains the earliest musical division of a canon in Western history.[4]
Because this book was written by a geometrician, all letter ratios and number ratios refer exclusively and unequivocally to the lengths of straight lines, which in turn represent the lengths of canon strings. Indeed, all ancient Greek[5] and Arabian[6]theorists who described the art of tuning from the perspective of practicing musicians, tabulated their tuning data in either string length units, or in length ratios. The author of the Division of the Canon specifies diplasios, hemiolios, epitritos, and epogdoos in Propositions 6–9, 12–14, and 19–20; the latter two propositions describe the process of tuning a complete diatonic “double-octave” on canon strings.
The oldest extant departures from this practice occurred approximately 400 years later in the Manual of Harmonics by the mathematician Nicomachus of Gerasa (b. c. A.D. 60) and in a dialog entitled On Music, contained in the Moralia of the biographer Plutarch (c. A.D. 46 – c. 120). Before we discuss these exceptions, note that both texts are devoid of the mathematical techniques and procedural methods required to describe a complete tuning on a musical instrument. The writers only assign numeric values to the “octave,” “fifth,” “fourth,” and “whole tone,” without integrating these intervals into a mathematical description of a complete scale. Such accounts typically stem from theoreticians who are not practicing musicians, which probably explains why neither Nicomachus nor Plutarch contributed a single original tetrachord division to the history of music.
Nicomachus abandons the concept of length ratios in Chapter 6 of his Manual of Harmonics. He begins the discussion by telling a fallacious story in which Pythagoras (c. 570 B.C. – c. 500 B.C.) compares various weights suspended from the ends of strings and, thereby, discovers that the “octave,” “fifth,” and “fourth” vary directly with tension:
Quote I
{Pythagoras} found that the string stretched by the greatest weight produced, when compared with that stretched by the smallest, an octave. The weight on one string was twelve pounds, while that on the other was six pounds. Being therefore in a double ratio [2:1], it produced the octave, the ratio being evidenced by the weights themselves. Again, he found that the string under the greatest tension compared with that next to the string under the least tension (the string stretched by a weight of eight pounds), produced a fifth. Hence he discovered that this string was in a hemiolic ratio [3:2] with the string under the greatest tension, the ratio in which the weights also stood to one another. Then he found that the string stretched by the greatest weight, when compared with that which was next to it in weight, being under a tension of nine pounds, produced a fourth, analogous to the weights. He concluded, therefore, that this string was undoubtedly in an epitritic ratio [4:3] with the string under the greatest tension . . .[7] (Bold text, and text in braces mine. Text in parentheses and ratios in brackets in Levin’s translation.)
In short, Nicomachus claims that one may produce these three cardinal consonances of music by tensioning strings according to the following “weight ratios”:
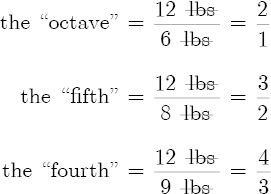
Nicomachus then assigns the following unspecified quantities to the “tonic,” “fourth,” “fifth,” and “octave” of the standard Greek Dorian Mode between E and E1:
Quote II
{Pythagoras} called the note partaking of the number 6, hypate {or E}, that of the number 8, mese {or A}, this number being in an epitritic proportion with the number 6; that of the number 9, he called paramese {or B}, which is higher than mese by a whole tone and what is more, stands in a sesquioctave {Latin for 9:8} proportion with it; that of 12, he called nete {or E1}. Filling out the intervening intervals in the diatonic genus with analogous notes, he thus subordinated the octachord to the consonant numbers, the double ratio [2:1], the hemiolic [3:2], the epitritic [4:3], and the difference between them, the sesquioctave [9:8].[8] (Italics, and text in braces mine. Ratios in brackets in Levin’s translation.)
If we assume that Nicomachus is conjuring “weight ratios,” then
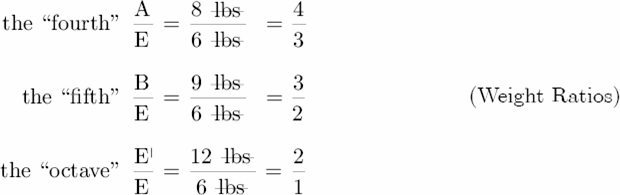
Contrary to observable fact, these weight measurements are false because Nicomachus assumed that “the notes,” or the aurally perceived frequencies of strings, are directly proportional to tension.
![]()
As discussed in Chapter 2, Section 6, the frequency of a string is directly proportional to the square root of tension (T), in pounds-force (lbf). Therefore, with all other variables held constant, to increase the fundamental frequency (F1) by an “octave,” we must increase Nicomachus’ tension of 6.0 lbf by the factor 2/1 squared. Similarly, to increase F1 by a “fifth” and by a “fourth,” we must increase the tension by the factors 3/2 squared and 4/3 squared, respectively:
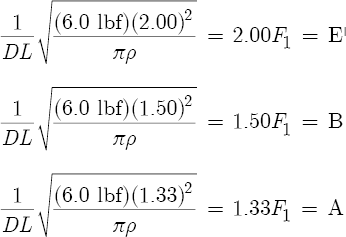
Therefore, the correct weights for these frequencies are
![]()
Nicomachus continues his numeric analysis of vibrating strings in Chapter 10, where he correctly concludes that frequency is inversely proportional to length. He begins the discussion by comparing functional differences in magnitude between the numeric values assigned to “weight ratios” and those assigned to length ratios:
. . . measurements based on the lengths . . . of strings . . . are seen to be inverse to measurements that are based on tension, [since] in . . . [the latter] case [1] the smaller the term, the lower the pitch, and the greater the term, the higher the pitch. [However], in the former case, there is an inverse proportion in that [2] the smaller the term, the higher is the pitch, while the greater the term, the lower is the pitch.[9] (Text and numbers in brackets mine.)
Observation 1 is true because lesser tension or a smaller term produces a lower tone, and greater tension or a larger term produces a higher tone. Furthermore, Observation 2 is also true because a shorter length or a smaller term produces a higher tone, and a longer length or a greater term produces a lower tone. Nicomachus then gives the following highly accurate description:
Quote III
If, therefore, one takes a long string that is kept under one and the same tension . . . — the string having been stopped by a bridge . . . at its very center so that the vibration caused by the plucking of the string may not progress beyond the half-way point — he will find the interval of an octave, the sound of half {hemiseias} the string compared with that of the whole string being in a greater proportion, that is, in a duple {2:1} proportion, a result exactly inverse to the reciprocal data of the length. And if one keeps the vibration down to a third of the string . . . the sound from two thirds {dimoirou} of the string will necessarily be in a hemiolic [3:2] relation to that of the sound from the whole string, or inversely proportional to the length of the string. And if one sections off a fourth part of the string . . . the sound from three parts of the string will be in an epitritic [4:3] relation to that of the whole string, or inversely proportional to the length of the string.[10] (Bold italics, and ratio in braces mine. Italics in braces in Nicomachus’ original text. Ratios in brackets in Levin’s translation.)
Refer to Equation 2.9 and observe that the frequency of a string is inversely proportional to length. Therefore, with all other variables held constant, to increase F1 by an “octave,” we must decrease a string length by the factor 2/1. Similarly, to increase F1 by a “fifth” and by a “fourth,” we must decrease the length by the factors 3/2 and 4/3, respectively. If we utilize Nicomachus’ numeric values to quantify string lengths, then
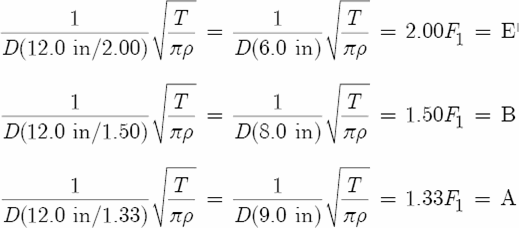
Although mathematicians in the 1st century A.D. had no scientific methods to determine the exact frequencies of strings, Nicomachus’ conclusions regarding rates of vibration are correct. He accurately observes that “the sound” from 1/2 of a string vibrates 2/1 times faster, from 2/3 of a string vibrates 3/2 times faster, and from 3/4 of a string vibrates 4/3 times faster than the whole length of the string. Therefore, if we utilize Nicomachus’ numeric values to quantify string vibrations (or modern frequencies, in cycles per second), then
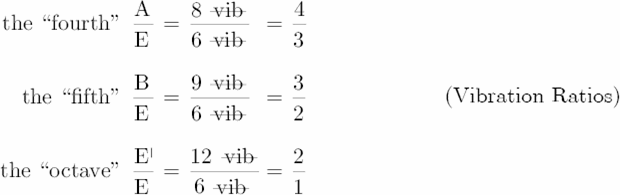
Note that for these musical intervals, “weight ratios” and “vibration ratios” are identical with respect to the numeric values of the numerators and denominators. Since “weight ratios” represent incorrect quantities, and “vibration ratios” represent correct quantities, we will avoid “weight ratios” in all future calculations. In other words, when Nicomachus and Plutarch refer to unspecified quantities such as 6, 8, 9, and 12, we will assume they mean vibration numbers that are equivalent to modern frequencies, such as 6.0 cps, 8.0 cps, 9.0 cps, and 12.0 cps. However, because “vibration ratios” are also problematic, we will only work with frequency ratios.
Finally, if we express these musical intervals according to the previously calculated string lengths, then
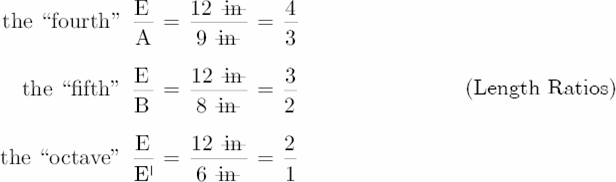
Of the Greek treatises on music that have survived, Quotes I and II represent the first occurrences where the terms diplasios, hemiolios, epitritos, and epogdoos do not refer to ancient length ratios, but to “weight ratios,” or to numerically identical “vibration ratios.” Consequently, whereas in the former case music theorists assign larger numbers to lower tones, in the latter case, they assign larger numbers to higher tones. In Sections 3.18–3.19 we will discuss how these two different quantifications of musical intervals affect the division of the “octave”; and in Section 3.20, we will examine several reasons why — throughout the history of music — creative theorists and musicians never mention weight numbers or vibration numbers in their descriptions and discussions of tetrachord divisions and scale constructions.
Section 3.18
Before we compare crucial differences between ancient length ratios and frequency ratios, let us first discuss several basic concepts of formal scale construction. A scale consists of a progression of tones expressed as a sequence of numbers in a definite order. The numbers contained in such a sequence are called terms. The Greeks defined three fundamental number sequences through the identification of three different means: the arithmetic mean, the harmonic mean, and the geometric mean. A mean represents an intermediate value, or several intermediate values, between two outer terms called the extremes. Consequently, in an arithmetic progression, in a harmonic progression, or in a geometric progression, a mean expresses a value that is larger than the small extreme, and smaller than the large extreme. In a musical context, a mean represents the division of a large interval into two or more smaller intervals. For the rest of this chapter, we will focus exclusively on Greek definitions of the arithmetic and harmonic means. See Chapter 9, Part I, and Chapter 10, Part III, for detailed discussions on geometric mean calculations.
The oldest extant Greek text that defines the three means of music is preserved in a fragment by Archytas (fl. c. 400 B.C.). Like Euclid (fl. c. 300 B.C.) after him, Archytas was a famous geometrician, who also wrote extensively on sound and music. Unfortunately, none of his works have survived intact. According to Ptolemy (c. A.D. 100 – c. 165), Archytas calculated three different tetrachord divisions in string length units, which means that Archytas used length ratios to identify the musical intervals of his scales.[11] Again, this is perfectly consistent with the work of a geometrician. We may calculate the three means with the following modern equations:
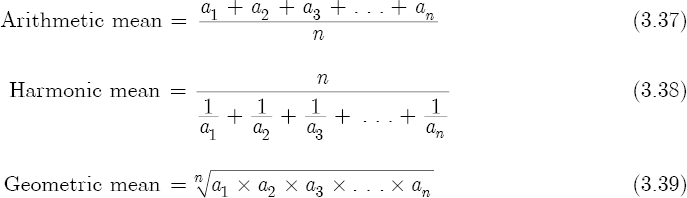
When there are only two variables (a, c), these equations simplify to

Archytas describes these three means in the following manner:
There are three means in music. One is arithmetic, the second geometric, the third subcontrary, which they call ‘harmonic’.
[1] There is an arithmetic mean when there are three terms, proportional in that they exceed one another in the following way: the second exceeds the third by the same amount as that by which the first exceeds the second. In this proportion it turns out that the interval between the greater terms is less, and that between the lesser terms is greater.
[2] There is a geometric mean when they are such that as the first is to the second, so is the second to the third. With these the interval made by the greater terms is equal to that made by the lesser.
[3] There is a subcontrary mean, which we call ‘harmonic’, when they are such that the part of the third by which the middle term exceeds the third is the same as the part of the first by which the first exceeds the second. In this proportion the interval between the greater terms is greater, and that between the lesser terms is less.[12] (Bold italics, bold text, and numbers in brackets mine.)
Because Archytas did not explicitly state whether the “greater terms” and “lesser terms” represent length numbers or vibration numbers, these definitions are completely open to interpretation. However, note carefully that in Definitions 1 and 3, the second or middle term “exceeds the third” term, which means that for the arithmetic mean and the harmonic mean, respectively, Archytas describes two descending sequences of numbers. As we shall see, in the context of musical interval calculations, music theorists primarily associate such progressions with string length measurements, or with the construction of length ratios.
The arithmetic mean refers to the familiar average value of two numbers. On the other hand, the harmonic mean is not so simple. Recall that Archytas’ definition specifies, “. . . that the part of the third [term] by which the middle term exceeds the third is the same as the part of the first [term] by which the first exceeds the second.” (Text in brackets mine.) Therefore, if the second term exceeds the third term by a given fractional value of the third term, the first term must exceed the second term by the same fractional value of the first term. Or, stated another way, the harmonic means is smaller than the large extreme by the same fraction that it is larger than the small extreme. For example, since in the progression 12:8:6, the harmonic mean (8) is smaller that the first term (12) by one third (1/3) of 12, the harmonic mean (8) must be larger than the third term (6) by one third (1/3) of 6:
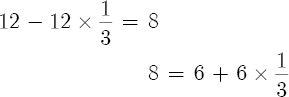
To understand how differences in length numbers and vibration numbers yield diametrically opposed interval divisions, begin by considering the arithmetic division of the “octave” according to length numbers. Refer to Equation 3.37A, substitute the values a = 12.0 in. and c = 6.0 in. into this equation, and calculate the arithmetic mean (ΛA) of the “octave”:
![]()
To compute the musical interval of the bridged canon string at 9.0 in. with respect to the fundamental at 12.0 in., divide the length ratio of the upper interval 12/9 by the length ratio of the fundamental 12/12:
![]()
And to calculate the musical interval of the bridged string at 9.0 in. with respect to the “octave” at 6.0 in., again divide the length ratio of the upper interval 12/6 by the length ratio of the lower interval 12/9:
![]()
Refer to Figure 3.22, and observe that we may interpret these interval ratios as the quantities described by Archytas’ first definition: the greater terms [12/9] identify the lesser arithmetic interval [4/3], and the lesser terms [9/6] identify the greater arithmetic interval [3/2].
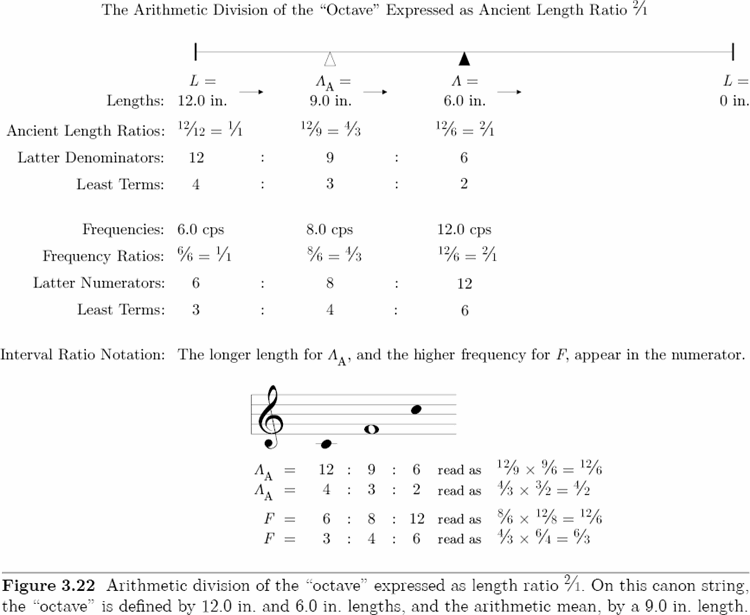
However, before we continue, consider a harmonic division of the “octave” according to vibration numbers. Refer to Equation 3.38A, substitute the values a = 6.0 cps and c = 12.0 cps into this equation, and calculate the harmonic mean (FH) of the “octave”:
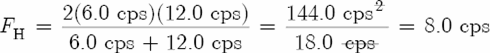
Again, examine Figure 3.22 and note that length numbers in arithmetic progression (12.0 in., 9.0 in., and 6.0 in.) produce vibration numbers in harmonic progression (6.0 cps, 8.0 cps, and 12.0 cps). Consequently, with respect to the latter vibration numbers, we may equally well interpret these frequencies as producing interval ratios described by Archytas’ third definition: the greater terms [12/8] identify the greater harmonic interval [3/2], and the lesser terms [8/6] identify the lesser harmonic interval [4/3].
So, although I describe Figure 3.22 as the arithmetic division of the “octave” expressed as length ratio 2/1, most scholars of ancient Greek music interpret Figure 3.22 as the harmonic division of the “octave” expressed as frequency ratio 2/1.
Next, consider a harmonic division of the “octave” according to length numbers. Refer to Equation 3.38A, substitute the values a = 12.0 in. and c = 6.0 in. into this equation, and calculate the harmonic mean (ΛH) of the “octave,”

Now, to compute the musical interval of the bridged canon string at 8.0 in. with respect to the fundamental frequency divide
![]()
Similarly, to compute the musical interval of the bridged string at 8.0 in. with respect to the “octave” at 6.0 in., divide
![]()
Refer to Figure 3.23, and observe that we may interpret these interval ratios as the quantities described by Archytas’ third definition: the greater terms [12/8] identify the greater harmonic interval [3/2], and the lesser terms [8/6] identify the lesser harmonic interval [4/3].
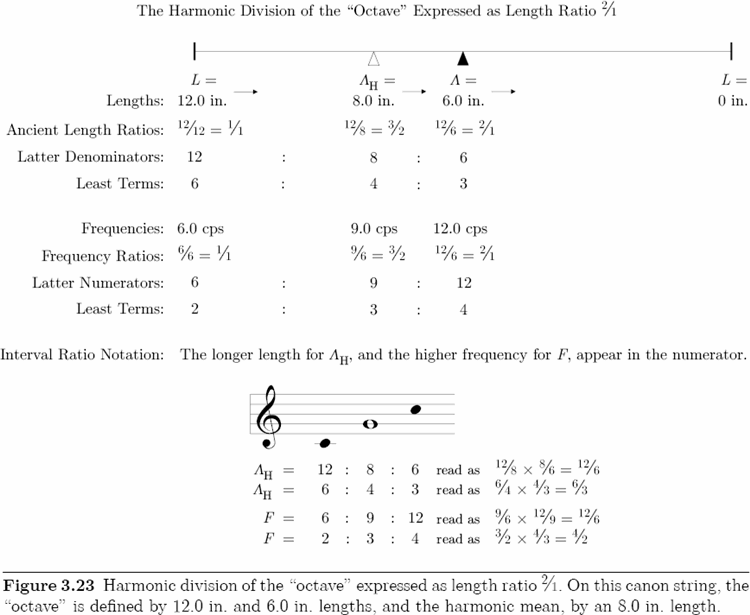
However, before we continue, consider an arithmetic division of the “octave” according to vibration numbers. Refer to Equation 3.37A, substitute the values a = 6.0 cps and c = 12.0 cps into this equation, and calculate the arithmetic mean (FA) of the “octave”:
![]()
Again, turn to Figure 3.23 and observe that length numbers in harmonic progression (12.0 in., 8.0 in., and 6.0 in.) produce vibration numbers in arithmetic progression (6.0 cps, 9.0 cps, 12.0 cps). Consequently, with respect to the latter vibration numbers, we may equally well interpret these frequency ratios as producing interval ratios described by Archytas’ first definition: the greater terms [12/9] identify the lesser arithmetic interval [4/3], and the lesser terms [9/6] identify the greater arithmetic interval [3/2].
So, although I describe Figure 3.23 as the harmonic division of the “octave” expressed as length ratio 2/1, most scholars of ancient Greek music interpret Figure 3.23 as the arithmetic division of the “octave” expressed as frequency ratio 2/1.
However, to confirm the historic authenticity of my interpretations as shown in Figures 3.22 and 3.23, see Chapter 10, Section 8, Quote I, and Chapter 10, Section 16, Quote II, for Ptolemy’s descriptions of the arithmetic and harmonic divisions of the “octave” on canon strings.
In Figure 3.22, notice that we may express interval ratios 4/3 and 3/2 with respect to string lengths, and interval ratios 4/3 and 6/4 [3/2] with respect to frequencies, in shorthand notation 4:3:2. Similarly, in Figure 3.23, we may express interval ratios 6/4 [3/2] and 4/3 with respect to string lengths, and interval ratios 3/2 and 4/3 with respect to frequencies, in shorthand notation 2:3:4. Now, observe carefully that the integers of the latter two sequences are identical. To distinguish between intervals based on string lengths on the one hand, and frequencies on the other, music theorists (who understand this crucial difference) use two different methods of notation. Length ratios appear in descending numerical order; here the first integer represents the overall length of an open string. Consequently, one writes interval ratios according to string length calculations as 4:3:2, 6:4:3, etc. In contrast, frequency ratios appear in ascending numerical order; here the first integer represents the fundamental frequency of an open string. Consequently, one writes interval ratios according to frequency calculations as 2:3:4, 3:4:6, etc.
Finally, as discussed in Chapter 10, Part VI, the arithmetic division of strings plays an extremely important role in determining the minor tonality, and the harmonic division, in determining the major tonality of Western music. Furthermore, although the musical intervals in Figures 3.22 and 3.23 are notated in ascending order, music theorists have also described the arithmetic and harmonic divisions of musical intervals in descending order. For example, in Chapter 10, Sections 62–64, we will examine the work of Jean-Philippe Rameau (1683–1764), who investigated a so-called subharmonic series, where musical intervals appear in reverse order from the harmonic series. Even though Rameau eventually conceded that such a phenomenon does not exist in nature, he nevertheless used a purely mathematical construction of the subharmonic series to define the minor tonality. Note, therefore, that the subject of interval notation is extremely complex, and that we must be constantly aware of the mathematical context in which a musical description of interval ratios occurs.
Section 3.19
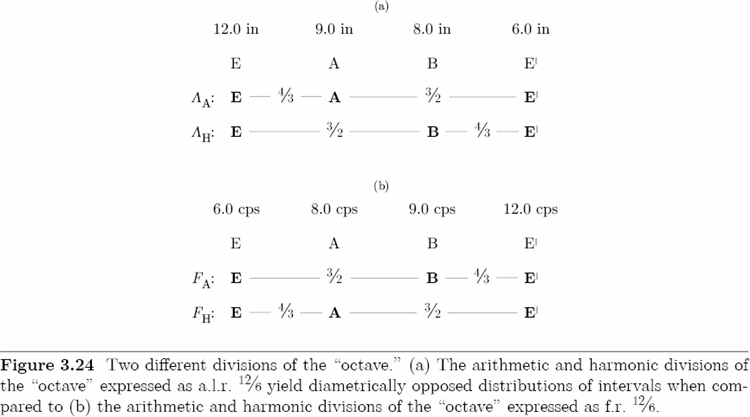
As dimensionless entities, ancient length ratios and modern frequency ratios are indistinguishable. For example, it makes no difference if a theorist specifies a.l.r. 4/3 or f.r. 4/3 because both ratios represent the “fourth.” Crucial differences occur only in the context of interval divisions! Figure 3.24 shows that the arithmetic division of the “octave” expressed as a length ratio yields a “fourth” as the lower interval, and a “fifth” as the upper interval; whereas the harmonic division yields a “fifth” as the lower interval, and a “fourth” as the upper interval. In contrast, an arithmetic division of the “octave” expressed as a frequency ratio yields a “fifth” as the lower interval, and a “fourth” as the upper interval; whereas the harmonic division yields a “fourth” as the lower interval, and a “fifth” as the upper interval.
We may also find evidence of the use of length ratios in Plato (427–347 B.C.). Although Plato mentions Philolaus (fl. c. 420 B.C.) on two occasions in a dialog entitled Phaedo,[13] he does not acknowledge the scientific and musical accomplishments of this renowned philosopher. (See Chapter 10, Section 10.) Consequently, he plagiarizes Philolaus’ famous diatonic tetrachord: 256/243, 9/8, 9/8 in a dialog entitled Timaeus. In commentaries by Nicomachus and Plutarch, both writers discuss the following passage from the Timaeus, which describes how God created the soul of the universe by dividing its divine “mixture” into several sequences of numerically defined musical intervals:[14]
And {God} proceeded to divide after this manner. First of all, he took away one part of the whole [1], and then he separated a second part which was double the first [2], and then he took away a third part which was half as much again as the second and three times as much as the first [3], and then he took a fourth part which was twice as much as the second [4], and a fifth part which was three times the third [9], and a sixth part which was eight times the first [8], and a seventh part which was twenty-seven times the first [27]. After this he filled up the double intervals [that is, between 1, 2, 4, 8] and the triple [that is, between 1, 3, 9, 27], cutting off yet other portions from the mixture and placing them in the intervals, so that in each interval there were two kinds of means, the one {harmonic} exceeding and exceeded by equal parts of its extremes, the other {arithmetic} being that kind of mean which exceeds and is exceeded by an equal number. Where there were intervals of 3/2 and of 4/3 and of 9/8, made by the connecting terms in the former intervals, he filled up all the intervals of 4/3 with the interval of 9/8, leaving a fraction over, and the interval which this fraction expressed was in the ratio of 256 to 243. And thus the whole mixture out of which he cut these portions was all exhausted by him.[15] (Bold italics, and text in braces mine. Text and numbers in brackets in Jowett’s translation.)
If it were not for the cosmic “mixture,” it would be difficult to imagine how the process of taking parts, cutting portions, and quantifying mathematical intervals depicts anything but the work of a master musician at a canon. I am particularly intrigued by the verbs to take and to cut, which also occur in Euclid’s canon tuning description.[16] However, because Plato does not explicitly state whether his ratios express length numbers or vibration numbers, this passage (like Archytas’ fragment) is completely open to interpretation. Of course, neither Nicomachus nor Plutarch allude to Plato’s obvious references to canon tuning techniques. So, it is not surprising that both authors suppressed the mathematical possibility that the “intervals of 3/2 and 4/3” could have originated from harmonic and arithmetic divisions of the “octave” expressed as length ratio 12/6. Approximately 500 years after Plato wrote the Timaeus, Nicomachus and Plutarch attempted to eliminate length ratios in favor of frequency ratios because they undoubtedly believed that the concrete reality of geometry interfered with developments in “pure” mathematics. In Chapter 8, Nicomachus gives the following interpretation:
Explanation of the references to harmonics in the Timaeus
. . . Plato expressed himself as follows: ‘so that within each interval there are two means, the one superior and inferior to the extremes by the same fraction, the other by the same number. He (the Demiurge) filled up the distance between the hemiolic interval [3:2] and the epitritic [4:3] with the remaining interval of the sesquioctave [9:8].’
For the double interval is as 12 is to 6, but there are two means, 9 and 8. The number 8, however, in the harmonic proportion is midway between 6 and 12, being greater than 6 by one third of 6 (that is, 2), and being less than 12 by one third of 12 (that is, 4). …
The other mean, 9, which is fixed at the paramese degree {or B}, is observed to be at the arithmetic mean between the extremes, being less than 12 and greater than 6 by the same number (3).[17] (Italics, and text in braces mine. Text in parentheses and ratios in brackets in Levin’s translation.)
Refer to Figure 3.24, and note that because Nicomachus identifies abstract number 9 as an arithmetic mean that represents the tone B, he interprets Plato’s division of the “octave” expressed as frequency ratio 12/6.
Finally, here is Plutarch’s explanation:
So Plato, wishing to show in terms of the science of harmonics the harmony of the four elements in the soul [represented by the numbers 6, 8, 9, and 12] . . . presents in each interval two means of the soul, in accordance with the ratio of music. For it so happens that in music the consonance of the octave has two mean intervals. … Now the consonance of the octave is seen to be in the duple ratio; and this ratio, expressed in numbers, is illustrated by six and twelve . . . Six then and twelve being the extremes, the hypate {or E} . . . is represented by the number six, the nete {or E1} . . . by the number twelve. … it is evident that the mese {or A} will be represented by the number 8, the paramese {or B} by the number nine.[18] (Italics, and text in braces mine. Text and numbers in brackets in Einarson’s translation.)
Again, since Plutarch equates the tone A with abstract number 8, and tone B with abstract number 9, the former number represents the harmonic division, and the latter number represents the arithmetic division of the “octave” expressed as frequency ratio 12/6.
Section 3.20
Many ancient writers avoid dimensional descriptions of quantities such as 6, 8, 9, and 12, because a specific reference to a “weight ratio” or “vibration ratio” would have compelled them to define a new kind of complicated “length ratio.” For example, in Section 3.17, Quote III, Nicomachus states that the sound of the “fifth” — or the frequency ratio of the “fifth” — is in a hemiolic [3/2] relation with the whole string; consequently, the length of the “fifth” must be in a dimoiros [2/3] relation with the whole string.[19] In his next example, the sound of the “fourth” — or the frequency ratio of the “fourth” — is in an epitritic [4/3] relation with the whole string; consequently, the length of the “fourth” must be in a hypepitritic [3/4] relation with the whole string. Here already, Nicomachus fails to identify the complicated fraction that describes three-fourths of the string. Instead, he simply compares four parts to three parts and, thereby, equates ancient length ratio 4/3 with frequency ratio 4/3.
The introduction of weight numbers and vibration numbers instigated a double standard whereby music theorists silently interpreted ancient length ratios as “weight ratios” or “vibration ratios.” Since ancient length ratio n/x is indistinguishable from Nicomachus’ “vibration ratio,” a switch from a.l.r. n/x to f.r. n/x incurs no numeric changes, and a switch in the opposite direction from f.r. n/x to a.l.r. n/x also incurs no numeric changes. However, all readers of ancient texts, and of most interpretations of ancient texts, must remain vigilant with respect to interval divisions. Confusion, mistakes, and anachronisms will inevitably occur if a reader fails to recognize whether a particular writer is describing the division of a.l.r. n/x, or of f.r. n/x.
Creative theorist-musicians like Euclid (fl. c. 300 B.C.), Ssu-ma Ch’ien (c. 145 B.C. – c. 87 B.C.), Ptolemy (c. A.D. 100 – c. 165), Al-Kindi (d. c. 874), Al-Farabi (d. c. 950), Ibn Sina (980–1037), Safi Al-Din (d. 1294), Ramis (c. 1440 – c. 1500), Ramamatya (fl. c. 1550), Zarlino (1517–1590), and Narayana and Ahobala (fl. c. 1670) reject or simply ignore “weight ratios” and “vibration ratios.” The inclusion of such ratios would have hopelessly confused their monumental achievements. In Chapters 10 and 11, we will examine detailed tuning descriptions by all these writers who — within the realm of human experience — based their work on the premise that frequency ratios are a function of length ratios.
[1]See Chapter 10, Section 58.
[2]Liddell, H.G., and Scott, R. (1843). A Greek-English Lexicon, p. 623. The Clarendon Press, Oxford, England, 1992.
[3](a) D’Ooge, M.L., Translator (1926). Nicomachus of Gerasa: Introduction to Arithmetic, p. 299. The Macmillan Company, New York.
(b) A Greek-English Lexicon, p. 1857.
[4](a) Barbera, A., Translator (1991). The Euclidean Division of the Canon: Greek and Latin Sources, p. 60. University of Nebraska Press, Lincoln, Nebraska.
The long version includes the text that spans pp. 170–185, which describes the tuning of a diatonic “double-octave.” See Chapter 10, Section 11, for a complete analysis and discussion of this tuning.
(b) Barker, A., Translator (1989). Greek Musical Writings, Volume 2, pp. 205–207. Cambridge University Press, Cambridge, Massachusetts.
In Barker’s translation, the long version includes Propositions 19 and 20.
[5]See Chapter 10, Part II.
[6]See Chapter 11, Part IV.
[7]Levin, F.R., Translator (1994). The Manual of Harmonics, of Nicomachus the Pythagorean, p. 84. Phanes Press, Grand Rapids, Michigan.
[8]Ibid., p. 85.
See Chapter 10, Figure 10, which shows the Greek note names hypate, mese, paramese, and nete in the context of a “double-octave” scale known as the Greater Perfect System.
[9]Ibid., p. 141.
[10](a) The Manual of Harmonics, p. 141.
(b) Jan, Karl von, Editor (1895). Musici Scriptores Graeci, p. 254. This work includes the Greek text of Nicomachus’ Manual of Harmonics. Lipsiae, in aedibus B.G. Teubneri.
In contrast to the discussion in this section on Greek terms for ratios whose quotients are less than one, Nicomachus uses hemiseias from hemisus which means half, and dimoirou from dimoiros which means two-thirds.
(c) Zanoncelli, L., Translator (1990). La Manualistica Musicale Greca, p. 164, 166. Angelo Guerini e Associati, Milan, Italy.
This book gives the Greek text of Nicomachus’ Manual of Harmonics as it appears in Jan’s Musici Scriptores Graeci, and it includes a modern Italian translation.
[11](a) Diels, H. (1903). Die Fragmente der Vorsokratiker, Griechisch und Deutsch, Volume 1, p. 428. Weidmannsche Verlagsbuchhandlung, Berlin, Germany, 1951.
Diels identifies Archytas’ description of three tetrachord divisions as authentic fragment A16. This page gives Archytas’ fragment as it appears in Ptolemy’s original Greek text.
(b) Burkert, W. (1962). Lore and Science in Ancient Pythagoreanism, pp. 379–380, Footnote 47. Translated by E.L. Minar, Jr. Harvard University Press, Cambridge, Massachusetts, 1972.
In Footnote 47, Burkert agrees with Diels that A16 is an authentic fragment. On p. 380, Burkert states, “. . . Archytas apparently assigned the smaller number to the high tone and the larger to the low.”
(c) Düring, I., Translator (1934). Ptolemaios und Porphyrios über die Musik, pp. 46–47. Georg Olms Verlag, Hildesheim, Germany, 1987.
(d) Greek Musical Writings, Volume 2, pp. 43–44, and p. 304.
[12]Greek Musical Writings, Volume 2, p. 42.
[13]Hamilton, E., and Cairns, H., Editors (1963). The Collected Dialogues of Plato, p. 44. The Phaedo translated by H. Tredennick. Random House, Inc., New York, 1966.
[14]Greek Musical Writings, Volume 2, pp. 59–60, Footnote 17.
Barker gives a complete analysis of these sequences.
[15](a) The Collected Dialogues of Plato, pp. 1165–1166. Quotation from the Timaeus translated by B. Jowett.
(b) Greek Musical Writings, Volume 2, pp. 59–60.
[16]See Chapter 10, Section 11.
[17]The Manual of Harmonics, p. 107.
[18]Einarson, B., Translator (1967). On Music, by Plutarch, pp. 401–403. In Plutarch’s Moralia, Volume 14. Harvard University Press, Cambridge, Massachusetts.
[19]See Footnote 10(b).