ON THE ART AND SCIENCE OF ACOUSTIC INSTRUMENTS
© 2000–2025 Cris Forster
For background information on the Greek prefix epi, on Equations 3.37A and 3.38A,
and on the arithmetic and harmonic divisions of musical intervals, see
Origins of Length Ratios.
CHAPTER 10: WESTERN TUNING THEORY AND PRACTICE
Part VI: Just Intonation
Section 10.38
Although Ramis managed to integrate 5-limit ratios into the 12-tone scale, he did not develop a theory of consonance. In 1558, seventy-six years after the publication of Ramis’ monochord, Gioseffo Zarlino (1517–1590) published a work entitled Istitutioni harmoniche in which he proposed (1) that the numero Senario (from the Latin: senarius, lit. composed of six in a group; fig. the number Series 1–6) constitutes the source of all possible musical consonances, and (2) that the harmonic division of strings expresses the “nature of Harmony” and produces “the consonances which the composers call perfect.” (See Section 10.44, Quote V.) However, at the end of his life, in the De tutte l’opere del R.M. Gioseffo Zarlino da Chioggia… edition of the Istitutioni (1589),[1] Zarlino argued that the arithmetic division and the harmonic division of the “fifth,” length ratio 3/2, are equally important to the development of music theory. He thereby became the first European music theorist to mathematically define what we now call the minor tonality and the major tonality, respectively, of musical composition.
In the Istitutioni harmoniche (1573), Zarlino acknowledges a theoretical contribution by the Arabian physician, scientist, and music theorist Avicenna, or Ibn Sina (980–1037), and plagiarizes a mathematical contribution by the German mathematician Michael Stifel (1487–1567). Zarlino first quotes a crucial sentence from a Latin translation of a compendium of Ibn Sina’s writings entitled Auicene perhypatetici philosophi: ac medicorum facile primi opera in luce redacta…, published in 1508.[2] Later in the text, Zarlino utilizes a harmonic division of a “double-octave and a fifth,” ratio 6/1, into five interval ratios as described in Stifel’s Arithmetica integra, published in 1544.[3] Although in the Dimostrationi harmoniche (1571),[4] Zarlino severely criticizes Stifel’s geometric division of the “tone,” ratio 9/8, two years later he fails to acknowledge Stifel’s stunning mathematical solution for the division of musical intervals into two or more harmonic means.
To understand Zarlino’s theory of musical consonance, the reader should thoroughly read Chapter 3, Sections 14–19, and carefully study Chapter 3, Figures 22 and 23. These two illustrations show the notation of length ratios and interval ratios in the context of the arithmetic division (ΛA), and the harmonic division (ΛH) of the “octave” on canon strings. Throughout Zarlino’s treatises, all ratios represent either (1) length ratios, or (2) interval ratios between length ratios. This means that frequency ratios, or interval ratios between frequency ratios, do not exist in his works.[5] The great Arabian theorist, Al-Farabi (d. c. 950), deliberately set the standard for notating musical ratios as expressions of string length measurements when he stated
The largest extreme of an interval, that which corresponds to the largest number, is, for certain mathematicians of another time, its lower extreme; for others, it is the upper {extreme}. In our opinion, it matters little, either from the point of view of theory or from the point of view of the ear, whether the large extreme is placed at the lower note or at the upper note. But having regarded up to now the lower note of an interval as being its large extreme, we will abide by this convention; besides, it is suited to the principles we stated, and facilitates our explanation of the rules of music; for it relates the measurement of the notes to the lengths [of strings] from which they come. The longest has the largest measurement and produces the lowest note; the shortest, which has the smallest measurement, gives the highest note.[6] (Text in braces mine. Text in brackets in La Musique Arabe.)
Therefore, one finds that in the musical treatises of the Arabian Renaissance,[7] all ratios refer exclusively to length ratios, or to interval ratios between length ratios.
During the 16th century, many European artists, scientists, and intellectuals were inspired to observe the physical processes and functions of nature. The Italian term la scienza naturale (lit. the science of natural things; in other words: physics), or the simplified expression la naturale (lit. the Natural), signified this renewed interest in natural phenomena. Consequently, there arose a heated debate between those who based their studies on mathematics, or abstract truth, and those who based their studies on physics, or concrete truth. The former philosophical approach is known as rationalism, and the latter, as empiricism.[8] In the Istitutioni harmoniche, Prima Parte, Cap. 19, Zarlino maintains that numbers have the capacity to manifest themselves as parts of “sounding bodies,” and that only through the quantifications of “sonorous numbers” are human beings able to comprehend the tones and ratios produced by vibrating strings. However, Zarlino resolves the debate between the science of numbers vs. the science of nature in the following chapter heading and passage from Prima Parte, Cap. 20:
The reason why music is the subordinate of arithmetic, and the
intermediary between la Mathematica [mathematics]
and la Naturale [physics].
. . . I am so bold to assert, that music is not only the subordinate of mathematics, but also of physics, not with respect to numbers, but with respect to tone, which is something natural. From it arises every modulation, every consonance, every harmony, and every melodic song. Avicenna [Ibn Sina], who also advocates such an interpretation, says: “Music derives its principles from the science of nature [physics] and the science of numbers [mathematics].”[9] (Text in brackets mine. Italics in Zarlino’s original text.)
Zarlino here quotes Ibn Sina from a chapter (or book) entitled Sufficientia (lit. Physics, or Science of Nature).[10] In short, Ibn Sina and Zarlino refused to compromise and, thereby, focused their attention on the best of both worlds. Given the personal nature and emphatic tone of his resolve, it seems to me that Zarlino was greatly influenced not only by Ibn Sina, but by other Arabian writers as well.
Section 10.39
To substantiate this assumption, let us prepare an examination of the Istitutioni harmoniche by first considering the division of musical intervals on canon strings in Ibn Sina’s Kitab al-shifa’ (Book of the cure) and in a treatise entitled Risalat al-Sharafiya fi’l-nisab al-ta’lifiya (The Sharafian treatise on musical conformities in composition) by Safi Al-Din (d. 1294). (See Section 10.36.) H.G. Farmer describes the former treatise by stating
This great work by the famous Avicenna — as he was known to the wide world — contained the entire sum of knowledge in science and philosophy known in Islamic lands, if not western Europe also. It includes a chapter (fann) on music which is divided into six discourses (maqalat) dealing respectively with the physics of sound, musical intervals, genres and species of melody, systems, and mutations, as well as rhythm and composition.[11]
Ibn Sina describes the arithmetic and harmonic division of the “octave” by noting
The octave is called the interval of absolute consonance [homophonic interval]; the fifth and the fourth are called intervals of similar notes [symphonic intervals]; sometimes one attributes to them the characteristic of inversion. The extreme degrees of the octave are, as we have said, [equivalent]; this is a special characteristic of that interval. The property of the two medium intervals is to compose an octave; the latter interval then contains between its two extreme terms an arithmetic mean and a harmonic mean. The ratio of the octave is, in fact, that of 4 to 2; if we introduce the number 3 between these two terms, we obtain two consecutive ratios [4:3:2] resulting from an arithmetic mean term. The ratio of the two largest terms is that of the fourth [4:3], and the ratio of the two smallest, that of the fifth [3:2]. Moreover, the ratio of 6 to 3 also constitutes an octave ratio; if between these two terms we introduce a third, i.e., 4, we obtain two consecutive ratios [6:4:3] resulting from a harmonic mean term. The ratio of the two largest terms is that of the fifth [6:4], and of the two smallest, that of the fourth [4:3]. The two ratios of the fourth and of the fifth form a replica [or form an “octave” with] one another, when they have a common degree, and when they are arranged in opposite directions. Take, for example, a fourth; it has an upper degree [3] and a lower degree [4]. If its upper degree also belongs to an interval of a fifth [3:2] of which it constitutes the low note [3], that is to say, if it [3] is followed by another higher note whose value is equal to 2/3 of its own [that is, 3 × 2/3 = 2], by playing the common note [3] and the uppermost note [2], [or 3:2], followed by the common note [3] and the lowest note [4], [or 4:3], the ear will perceive the same sensation. It will be the same if one plays a fourth [4:3] going up and a fifth [3:2] going down. This happens because the ratio of the uppermost degree to the lowest is that of the octave.[12] (Bold italics, and text, ratios, and integers in brackets mine. Bold italics in brackets my correction of the “puissance” translation error in La Musique Arabe.)
Figure 10.34 shows that Ibn Sina’s ratios refer unequivocally to string length measurements. Although so-called Western theorists such as Nicomachus,[13] Theon,[14] and Boethius[15] (see Section 10.4) also described various arithmetic and harmonic divisions of the “octave,” Ibn Sina was the first music theorist to define both of these divisions in least terms. Notice that one cannot reduce Ibn Sina’s integers of the arithmetic division of the “octave” expressed as interval ratios 4:3:2, or the harmonic division of the “octave” expressed as interval ratios 6:4:3. As discussed below, it is extremely important to notate arithmetic and harmonic divisions in least terms when one attempts to divide a given length ratio into three or more interval ratios.
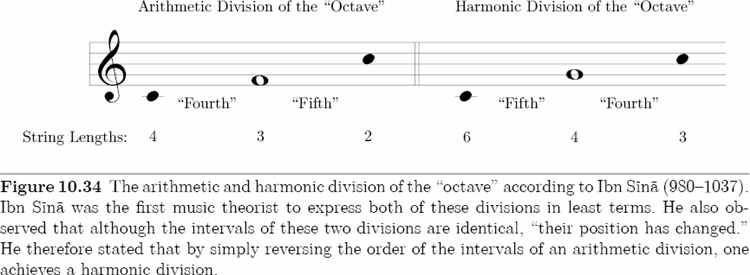
Ibn Sina also observed that the intervals produced by an arithmetic and a harmonic division of a given interval are identical:
Quote I
Now, we have already seen that in giving a ratio an arithmetic mean, we obtain two ratios identical to those that result from a harmonic mean; but their position has changed.[16] (Bold italics mine.)
Therefore, since the calculation of a harmonic mean is more difficult than an arithmetic mean, Ibn Sina offers the following convenient solution:
Quote II
When it comes to making this division by way of the harmonic mean, if we do not find any number [integer] that can serve this purpose, it will suffice to place in the lower [position] the largest of the two ratios obtained by way of the arithmetic mean.[17] (Bold italics, and text in brackets mine.)
Finally, consider this passage by Safi Al-Din, which constitutes the first known description of the arithmetic division of the “fifth”:
If we are then asked which are the two intervals whose ratios are made of consecutive numbers taken in the natural order of numbers, and which together exactly complete the interval of the ratio 1 + 1/2 [3:2], the fifth, the easiest way to solve the problem will be this: We double the term of this ratio that represents the higher note, that is 2, and we will thereby know that of the two intervals required, the one placed in the upper [position] will be in the ratio 1 + 1/4 [5:4], and the one placed in the lower [position] in the ratio 1 + 1/5 [6:5].[18] (Bold italics, and text and ratios in brackets mine.)
Figure 10.35 shows that the arithmetic division of length ratio 3/2 produces a “minor third,” ratio 6/5, as the lower interval, and a “major third,” ratio 5/4, as the upper interval. In least terms, we may notate this division as interval ratios 6:5:4.

Section 10.40
Zarlino introduces the concept of his Senario in Prima Parte, Cap. 15:
Quote I
On the characteristics of the numero Senario and its parts,
and the relations between them, one finds the form
of every musical consonance.
Although the numero Senario possesses many special characteristics, I will nevertheless, in order not to deviate, enumerate only those [properties] that are suitable for our purpose. First, it represents the first of the perfect numbers. It contains parts which stand in the following relation to one another: if one picks out two arbitrary parts, they always indicate the relation or the form of a proportion of a musical consonance — whether it concerns a simple or a compound consonance — as one can see in the following figure [see Figure 10.36].[19] (Text in brackets mine. Italics in Zarlino’s original text.)
In the original engraving that follows, Zarlino assigns traditional Latin names to the musical intervals included in the Senario. To discuss the mathematical significance of this illustration, Figure 10.36 expresses these interval names as ratios.
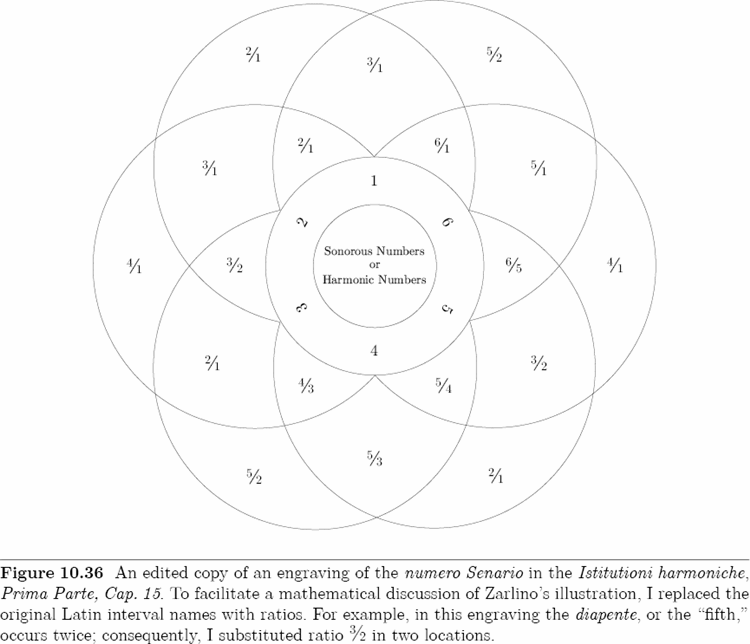
When viewed from a historical perspective, Quote I is of paramount importance to the development of European music because it shatters the 3-limit barrier of Pythagorean theory. As described in Section 10.5, the Pythagoreans recognized only five consonances: 4/1, 3/1, 2/1, 3/2, 4/3. These ratios represent all possible interval combinations in the series 1, 2, 3, 4. Figure 10.37 shows that one obtains these consonances through a division of a canon string into two, three, and four aliquot parts.

Dissatisfied with the limitations of only five consonances, Zarlino proposed the number six, or the first perfect number, as the underlying mathematical principle of all musical consonances. By definition, a perfect number is a positive number that equals the sum of its positive divisors. In this case, 1 + 2 + 3 = 6. To demonstrate the musical potential of the Senario, or of the series 1, 2, 3, 4, 5, 6, Zarlino continues his description with the arithmetic division of the “octave,” expressed as interval ratios 4:3:2, and the arithmetic division of the “fifth,” expressed as interval ratios 6:5:4. He then states that a “harmonic divisor,” or a harmonic division of these two ratios, would have placed the “parts,” or these interval ratios, in “reverse order.”
Quote II
Its parts [i.e., the six parts of the Senario] are so sequenced and arranged, that the forms of either of the two simplest and largest consonances [i.e., 2:1 and 3:2] — which the musicians call perfect because they are contained in the parts of the number 3 — may be divided by a middle number into two harmonically proportioned parts. First, one finds the octave, without an inner term, in the form and the relation of 2:1. Then the octave is divided by the number 3, which is situated between 4 and 2, into two consonant parts [4:3:2]; that is, into the fourth, which is between 4 and 3, and into the fifth between 3 and 2. One finds the fifth in turn between the numbers 6 and 4, which is divided by 5 into two consonant parts [6:5:4]; that is, a major third between 5 and 4, and a minor third, which is contained in the numbers 6 and 5.
I wrote that the parts are arranged according to a harmonic proportion. However, this does not concern the order of the proportions (because they are in reality in arithmetic order), [i.e., 4:3:2 or interval ratios 4:3, 3:2; and 6:5:4 or interval ratios 6:5, 5:4], but applies only to the relation of the parts as determined by the middle number. Because these parts exist in such large quantity and in as many relations as there are parts — which are fashioned [into proportions] by a middle number or a harmonic divisor — although in a reverse order, [i.e., 6:4:3 or interval ratios 3:2, 4:3; and 15:12:10 or interval ratios 5:4, 6:5], as we will see below in the appropriate place.[20] (Bold italics, and text and ratios in brackets mine. Text in parentheses in Fend’s German translation.)
In Section 10.42, we will discuss the mathematics of the latter paragraph in full detail. For now, we conclude that while it may be impossible to prove a direct connection between the musical treatises of Ibn Sina and Safi Al-Din on the one hand, and Quote II from Zarlino’s Istitutioni harmoniche on the other, we should not assume that the latter text states new or original ideas.
Section 10.41
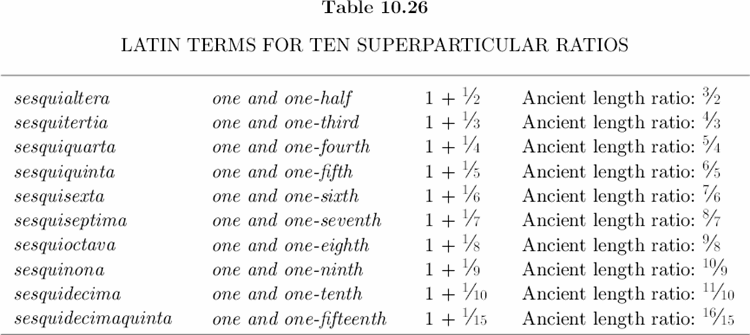
Before we continue this discussion on the arithmetic and harmonic divisions of length ratios, let us first examine Latin expressions for ratios, which appear in countless European treatises on music. Ordinarily, the Latin prefix sesqui means one and a half, or one-half more; consequently, the Latin word sesquiopus means the work of a day and a half, and sesquicentennial literally equals (100 years ÷ 2) + 100 years = 150 years. However, this simple definition of sesqui does not apply to mathematical descriptions of epimore or superparticular ratios. (See Section 10.4.) Although medieval and Renaissance theorists retained old Greek names of musical intervals such as diapente for “fifth,” diatessaron for “fourth,” ditone (ditonon) for “major third,” tone (tonon) for “whole tone,” etc., they substituted new Latin mathematical expressions such as sesquialtera for hemiolios [3/2], sesquitertia for epitritos [4/3], sesquiquarta for epitetartos [5/4], sesquioctava for epogdoos (epiogdoos) [9/8], etc. Except for the first example, note that the Latin prefix sesqui replaces the Greek prefix epi. To understand the derivations of these new constructions, refer to Table 10.25, which gives the Latin terms for the first ten ordinal numbers. Now, since sesqui means one-half more, and since alter means second — as in the second part of a whole, or one-half — sesquialtera literally equals (1/2 ÷ 2) + 1/2 = 3/4. However, turn to Table 10.26 and note that sesquialtera actually means 1 + 1/2 = 3/2; similarly, since tertius means third — as in the third part of a whole, or one-third — sesquitertia literally equals (1/3 ÷ 2) + 1/3 = 1/2; however, sesquitertia actually means 1 + 1/3 = 4/3. As a result, we conclude that in the context of musical ratios, the Latin prefix sesqui simply describes the operation of addition, which means that it has the identical mathematical function as the Greek prefix epi. (See Chapter 3, Section 17.) Consequently, sesquiquarta denotes one-fourth in addition, and connotes one and one-fourth: 1 + 1/4 = 5/4.
Finally, with respect to epimere or superpartient ratios, Table 10.27 lists the Latin terms of seven ratios that appear in Table 10.3. To identify a given ratio, extract the numerator and the denominator contained in the term. First, to determine the denominator, simply identify the last word. Second, to calculate the numerator, identify an inner value — bi for 2, tri for 3, quadri for 4, or quinque for 5 — and add this quantity to the denominator. Therefore, superquadripartiens-quinta describes a ratio with 5 in the denominator, and 5 + 4 = 9 in the numerator, or ancient length ratio 9/5.


Section 10.42
In preparation for a discussion on Zarlino’s arithmetic and harmonic division of length ratio 6/1 on canon strings, we must first review his methods of calculation. With respect to the first kind of division, Zarlino states in Prima Parte, Cap. 36,
For example, we want to arithmetically divide a sesquialtera, which is formed by the basic numbers 3 and 2. The former includes consecutive prime numbers, which must first be doubled. Then we obtain the numbers 6 and 4. When they are added, the result is 10; when this result is divided into two equal parts, the result is 5. Therefore, I say that 5 is the divisor of our proportion. Because it not only produces the same differences in this proportionality, but also divides the proportion (as is the characteristic of an arithmetic proportionality) into two unequal relations, in such a manner, that one finds between the larger numbers the smaller proportion, and inversely, between the smaller {numbers}, the larger {proportion}. The sesquiquinta {exist} between the 6 and 5, and the sesquiquarta, between the 5 and 4 . . . It is true, that one will refer to this [sequence of numbers] as a progression rather than a proportionality. Because one begins with the smaller number, comes to a middle {number}, and from this, to the larger {number}. One progresses with equal distances. One always finds unity or two or three or another number which produces the mentioned distance.[21] (Text in braces mine. Text in parentheses and brackets in Fend’s German translation.)
In short, after doubling the outer terms, Zarlino utilizes Equation 3.37a and calculates the arithmetic division of length ratio 3/2 in the following manner:

This arithmetic progression expresses an ascending sequence of musical intervals: a low “minor third,” length ratio 6/5, followed by high “major third,” length ratio 5/4.
With respect to the second kind of division, Zarlino states in Prima Parte, Cap. 39,
If we want to harmonically divide a sesquialtera, which is formed by the basic numbers 3 and 2, then we will first divide it arithmetically in the manner that I stated above. Then we obtain an arithmetic proportionality in the numbers 6:5:4. Second, to cause it to become a harmonic proportionality, we multiply the 6 and the 4 times the 5, and then [we multiply] the 6 times the 4. From the products we derive the desired [harmonic] division, which is formed by the numbers 30:24:20 . . . Because the relation between the numbers 6 and 4, which indicates the distance between the harmonic numbers, corresponds to that between the numbers 30 and 20. They are the outer terms of the sesquialtera, which are divided into a sesquiquarta between 30:24 [5:4], and into a sesquiquinta with the relation 24:20 [6:5]. Thus, one finds between the larger numbers the larger relation, and between the smaller [numbers], the smaller [relation], and this is the characteristic of this proportionality.[22] (Text and ratios in brackets mine.)
To summarize, Zarlino does not utilize Equation 3.38a, but calculates the harmonic division of length ratio 3/2 based on the three terms of the arithmetic division of 3/2.
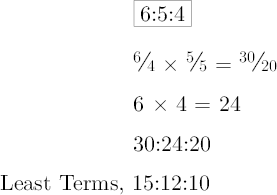
This harmonic progression expresses the former ascending sequence of musical intervals in “reverse order”: a low “major third,” length ratio 15/12 = 5/4, followed by high “minor third,” length ratio 12/10 = 6/5.
Section 10.43
Next, to prepare for Zarlino’s arithmetic and harmonic divisions, we must also consider the work of Michael Stifel. In commentaries to his German translation of the Prima Parte and Seconda Parte of the Istitutioni harmoniche, Michael Fend gives the following description and translated excerpt from Stifel’s Arithmetica integra (1544):
Zarlino owes the realization of the reciprocity of both sequences of ratios indirectly to Arithmetica integra, by Michael Stifel who . . . first taught how harmonic sequences can be constructed beyond three terms provided that one derives them from arithmetic sequences. Stifel began with the observation that a cube, which possesses 6 faces, 8 vertices, 12 edges, and 24 face-angles, represents a harmonic proportion, and he transferred this sequence of proportions (6:8:12:24) to a descending sequence of tones: dd, aa, d, D. He compared it to the [descending] sequences cc, gg, c, C; aa, e, a, A; g, d, G, Γut [lit. Gamma ut, or lowest G], and then unexpectedly advanced the thesis that one
‘can produce — from any given arithmetic progression — a harmonic [progression], which includes as many terms as the arithmetic [progression]. One proceeds in the following manner: Multiply the terms of your arithmetic proportion in sequence with one another. Then divide the product by the individual terms of your arithmetic progression, beginning with its largest term. From the arithmetic sequence 1, 2, 3, 4, 5, 6 comes the harmonic [sequence] 10, 12, 15, 20, 30, 60. In the same manner, out of a given harmonic sequence comes an arithmetic [sequence]!’ [23] (Bold italics and text in brackets mine. The harmonic progression in parentheses in Fend’s German commentary.)
Regarding Stifel’s cube analysis, refer to Equation 3.38a, and for a given string where a = 6 units and c = 12 units, calculate ΛH = 8 units; and where a = 8 units and c = 24 units, ΛH = 12 units. Consequently, Stifel’s harmonic progression demonstrates that the “double-octave,” ratio 24/6 = 4/1, has two harmonic means, namely, 8 and 12. Now examine Figure 10.38, which shows Stifel’s descending tones: dd, aa, d, D, on a canon string with a length of 6 units, 8 units, 12 units, and 24 units, respectively.

Because Stifel interprets the harmonic progression 6:8:12:24 as a descending sequence of tones, we must, in turn, interpret his series as a descending sequence of interval ratios: 8/6, 12/8, 24/12, or simply 4/3, 3/2, 2/1, respectively. Now, turn to Chapter 3, Figure 12, and observe that if we play these three interval ratios in ascending order: 2/1, 3/2, 4/3, Stifel’s harmonic division of the “double-octave” produces the first three intervals of the harmonic series, namely, the “octave,” “fifth,” and “fourth.”
With respect to the transformation of the arithmetic progression 1, 2, 3, 4, 5, 6 into the harmonic progression 10, 12, 15, 20, 30, 60, Stifel calculated the integers of the latter sequence in three steps:

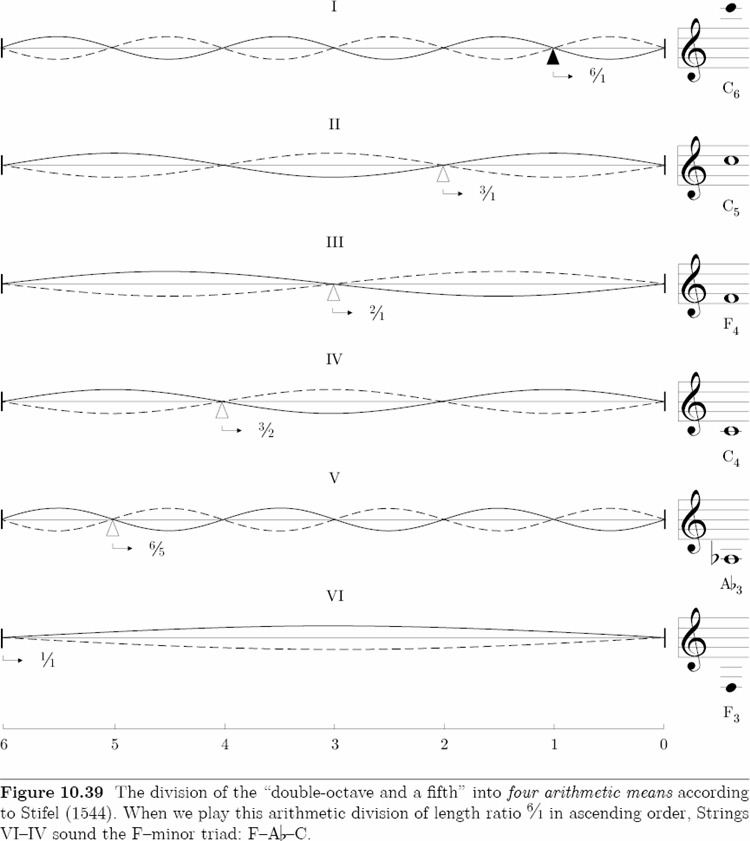
Step 3 reduces the quotients of Step 2 to least terms. Although Stifel did not explicitly interpret the arithmetic and harmonic division of the “double-octave and a fifth,” ratio 6/1, as a descending sequence of tones, it is highly likely that he also experienced these two divisions on canon strings. Figure 10.39 shows the arithmetic progression 1:2:3:4:5:6 as a descending sequence of tones: C6, C5, F4, C4, Ab3, F3, or a descending sequence of interval ratios: 2/1, 3/2, 4/3, 5/4, 6/5. If we express each tone as a length ratio, and play them in ascending order: F3–1/1, Ab3–6/5, C4–3/2, F4–2/1, C5–3/1, C6–6/1, we find that the first three tones produced by Strings VI–IV sound the F-minor triad: F–Ab–C. Finally, examine Figure 10.39 to see that this so-called arithmetic division actually consists of a sequence of increasing string lengths, where each succeeding length is an integer multiple of the first length. That is, String I has a length of 1 unit, String II has a length of 1 unit × 2 = 2 units, etc. Hence, Stifel’s descending division of ancient length ratio 6/1 into four arithmetic means: 1, 2, 3, 4, 5, 6.
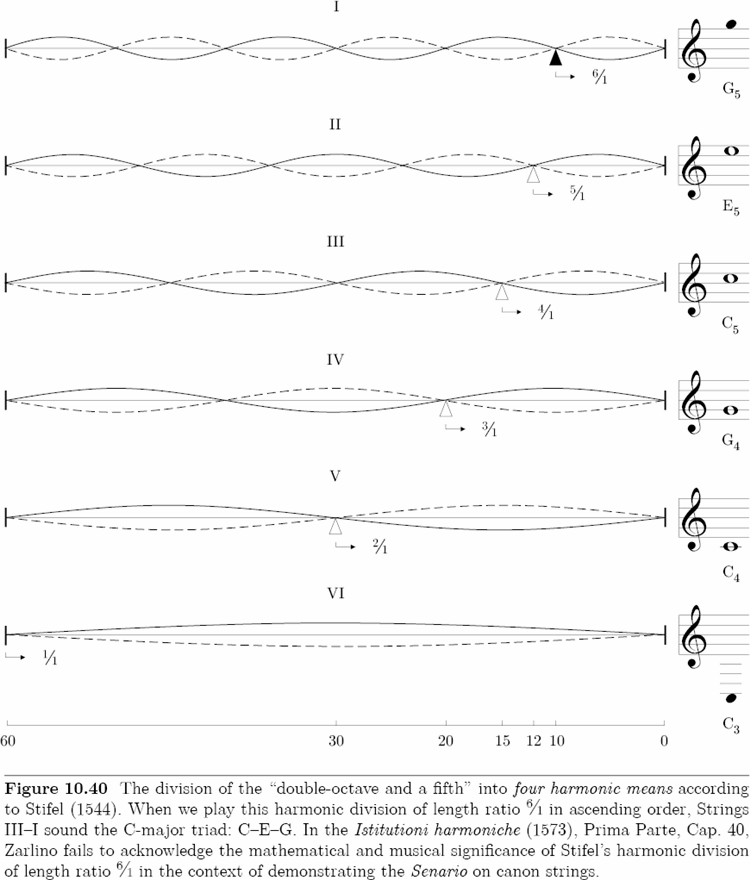
In contrast, Figure 10.40 shows Stifel’s harmonic progression 10:12:15:20:30:60 as a descending sequence of tones: G5, E5, C5, G4, C4, C3, or a descending sequence of interval ratios: 12/10, 15/12, 20/15, 30/20, 60/30, or simply 6/5, 5/4, 4/3, 3/2, 2/1, respectively. If we express these tones as length ratios, and play them in ascending order: C3–1/1, C4–2/1, G4–3/1, C5–4/1, E5–5/1, G5–6/1, we find that the last three tones produced by Strings III–I sound the C-major triad: C–E–G. Now, turn back to Chapter 3, Figures 10, 13, and 15, and observe that the standing waves in Figure 10.40 represent the first six modes of vibration of a flexible string. The first complete analysis of the mode shapes and mode frequencies of vibrating strings exists in a work entitled Système général des intervalles des sons… by Joseph Sauveur (1653–1716), published in 1701. (See Section 10.56.) We conclude, therefore, that Stifel comprehended the mathematical and musical significance of the division of ancient length ratio 6/1 into four harmonic means: 10, 12, 15, 20, 30, 60, approximately 150 years before Sauveur discovered the harmonic progression 1, 1/2, 1/3, 1/4, 1/5, 1/6, . . . as a natural phenomenon of subdividing strings.
Section 10.44
In the Istitutioni harmoniche, Prima Parte, Cap. 40, Zarlino begins his formal demonstration of the Senario on canon strings by first summarizing the fundamental differences between the “compound unities” of the arithmetic division, and the “sonorous quantities” of the harmonic division.
The harmonic proportionality possesses the same proportions as the arithmetic [proportionality] because the forms of the consonances are contained (as we saw) in the parts of the numero Senario; however, in the case of the arithmetic proportionality, among the smaller numbers exist the larger proportions, and among the larger [numbers], the smaller [proportions]; while one finds the opposite in the case of the harmonic proportionality, that is, we have among the larger numbers the larger proportions, and among the smaller [numbers], the smaller [proportions]. This difference stems from the fact that the one [the former] is associated with pure numbers, and the other [the latter], with sonorous quantities. They progress in opposite directions, that is, one [the former] increases, the other [the latter] decreases in relation to their respective starting point, as I showed. None of them deviates from the natural progression, which one finds in the order of the proportions. This [order] is formed by the numbers in the following manner: in the arithmetic proportionality, the numbers form compound unities, while in the harmonic proportionality, they are parts of sonorous quantities.[24] (Bold italics, and text in brackets mine. Italics in Zarlino’s original text. Text in parentheses in Fend’s German translation.)
As discussed in Section 10.43, the “compound unities” represent increasing string lengths that are integer multiples of the first string length, and the “sonorous quantities” represent decreasing string lengths of a manually subdivided string. Zarlino then continues with the arithmetic division of an “octave and a fifth,” or length ratio 3/1.
Quote III
In order to better understand these things, we will give an example. We draw a line AB, which for an arithmetician represents unity, and for a musician, a sonorous body, hence a string. Its length is one foot. If we want to give it an arithmetic progression, then we must leave it whole and undivided, because one may not divide unity of an arithmetic progression. Thus an [arithmetic] proportion, consisting of three numbers, is given in such a manner, that the proportion of a tripla [3:1] is divided by a mean into two parts.
We must proceed in the following manner: First, the mentioned line (if possible) is to be doubled, so that unity is doubled [to form] a duality, which follows unity directly. After we doubled it, we have the line AC of a two-foot length. If we compare the doubled line AC with the line AB, then we discover between them the proportion of the dupla [2:1], which is first in the natural order of the proportion, as one also finds between the numbers two and one. When we want to find the third term in this kind of progression, we must extend the line AC to a three-foot length, so that it reaches the point D, because three directly follows two. Then we will have the proportion of the tripla between DA and BA, because AD is measured exactly three times by AB, or AD contains AB three times, as in the case of numbers the three contains the one three times. And the proportion from AC can be divided into two parts in the following manner: in a dupla CA and BA, and in a sesquialtera [3:2] DA and CA, indeed an arithmetic proportionality . . .[25] (Ratios in brackets mine.)
At this point in the text, Zarlino includes a simple figure to illustrate his division on a canon string, but he neither describes nor demonstrates the arithmetic division of number six, length ratio 6/1, in full detail. As an alternative, refer to Figure 10.41, which takes Zarlino’s arithmetic method to its logical conclusion. Here Strings I–III illustrate the first three steps described by Zarlino in Quote III, and String III shows the complete arithmetic division of length ratio 3/1, interval ratios 3:2:1. The column to the left of the string gives Zarlino’s method for calculating the arithmetic mean, and the staff to the right of the string shows C5 as the arithmetic mean between C6 and F4. Finally, Strings IV–VI demonstrate three succeeding constructions that result in the arithmetic division of length ratio 4/2, interval ratios 4:3:2, of ratio 5/3, interval ratios 5:4:3, and of ratio 6/4, interval ratios 6:5:4.

Zarlino then continues with the harmonic division of length ratio 6/2 [3/1].
Quote IV
However, if we want to construct a harmonic progression, we will proceed in the following manner: First, we divide the mentioned line AB at its center, the point C, because the half comes before every other part. I now say that one finds between the given string AB and its half CB . . . the proportion of the dupla [2:1], which is the first in the natural order of the proportions. Then we will decrease the mentioned line AB by 2/3 at the point D, and we will thus obtain the proportion of the sesquialtera [3:2], which takes the second place in the order of the proportions. I say that the sesquialtera exists between CB and DB, furthermore the tripla [3:1] [exists] between AB and DB, which are [both] divided by CB into two proportions according to the harmonic proportionality . . .[26]
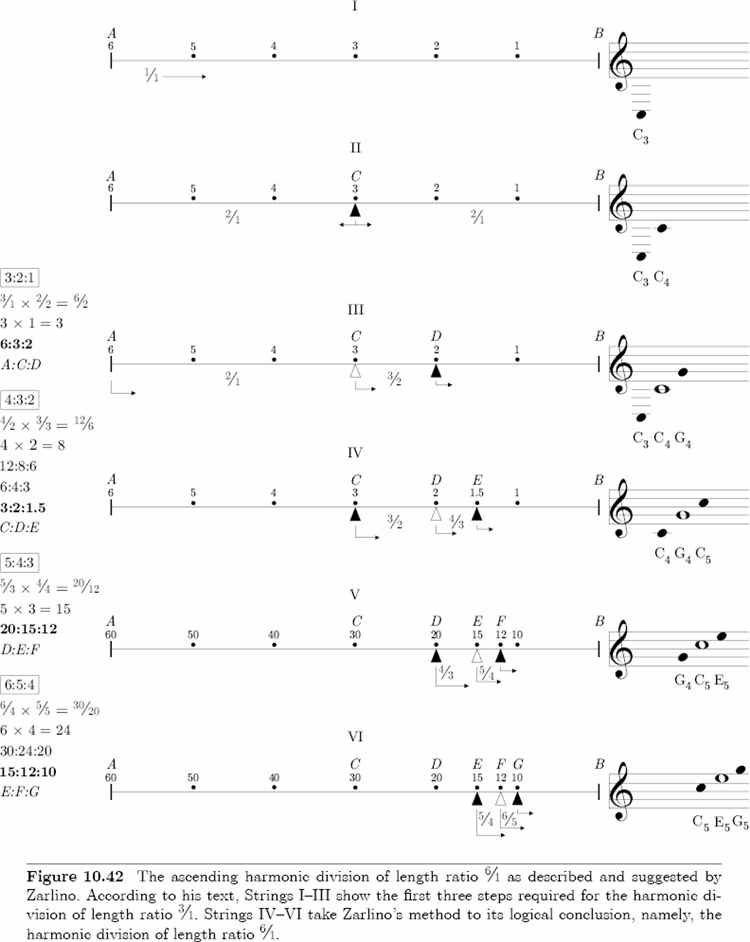
Here again, Zarlino includes a simple figure to illustrate his division on a canon string, but he neither describes nor demonstrates the harmonic division of length ratio 6/1 in full detail. So, refer to Figure 10.42, which takes Zarlino’s harmonic method to its logical conclusion. Strings I–III illustrate the first three steps described by Zarlino in Quote IV, and String III shows the complete harmonic division of length ratio 6/2 [3/1], interval ratios 6:3:2. In conformity with Zarlino’s method of calculating a harmonic division based on the three terms of a corresponding arithmetic division, the column to the left of String III gives the latter three terms in a rectangular frame. Also, the staff to the right of the string shows C4 as the harmonic mean between C3 and G4. Finally, Strings IV–VI demonstrate three succeeding constructions that result in the harmonic division of length ratio 6/3 [2/1], interval ratios 6:4:3, of ratio 20/12 [5/3], interval ratios 20:15:12, and of ratio 30/20 [3/2], interval ratios 15:12:10. Note carefully the transition in string length units between Strings IV and V. Although it is possible to extend the fractional string length notation 3.0 : 2.0 : 1.5 of String IV, to 2.0 : 1.5 : 1.2 for String V, and to 1.5 : 1.2 : 1.0 for String VI, in Quote V below Zarlino explicitly gives Stifel’s harmonic progression 60:30:20:15:12:10 as the harmonic division of number six. Consequently, the mathematical complication that requires a transition from 6 units for the overall length of Strings I–III, to 60 units for the overall length of Strings IV–VI, probably explains why Zarlino neither described nor illustrated the complete harmonic division of length ratio 6/1.
Zarlino continues with the following comparison of the arithmetic and the harmonic division of length ratio 3/1:
And as the numbers of the arithmetic progression are multiplied unities, so those [numbers] of the harmonic [progression] represent the number of parts that can be determined from a sonorous body, [and] which originate from the subdivision of this body. Therefore, in the former one regards the multiplication of unity, as in the following sequence: 3:2:1. And in the latter, one regards the multiplication of parts on a divided object, which is formed by the numbers 6:3:2. Because if we regard the whole divided into its parts, then we discover that the line CD is the smallest part of the line AB, and that it measures AB altogether six times, the line CB, three times, and the line DB, two times.[27] (Text in brackets mine.)
With respect to these two different kinds of divisions on canon strings, Zarlino concludes
Quote V
Now it can be seen, that in the harmonic progression [6:3:2], the larger numbers [6:3] contain the larger proportions and the lower sounds [i.e., the “octave”], while the smaller numbers [3:2] correspond to the smaller proportions and the higher sounds [i.e., the “fifth”]. Because they [the higher sounds] are brought forth on strings with smaller dimensions, while in the case of the lower tones, the strings have larger dimensions. Furthermore, we can see: As one progresses in the arithmetic proportionality (provided that one would realize it in the manner shown) from the high to the low sound by multiplying the string length, so one proceeds in the harmonic [proportionality] in reverse from low to high by shortening the string. In the arithmetic progression [3:2:1] the intervals of the smaller proportion [3:2] have their position in the lower [sounds], [i.e., the “fifth”], contrary to the natura dell’Harmonia [lit. nature of Harmony], whose characteristic it is that the deep sounds possess a larger interval than the high [sounds], and these [the high sounds], in turn, [possess] a smaller [interval].
However, since all the proportions that belong to the arithmetic progression — because they follow the natural order of the proportions — also exist in the same order in the harmonic progression [that is, the “octave,” “fifth,” “fourth,”. . . descend in the arithmetic progression, and the “octave,” “fifth,” “fourth,”. . . ascend in the harmonic progression], we can now understand in which manner one should take the meaning of the words in Chapter 15, which state that in the terms of the numero Senario are contained all the forms of the simple musical consonances that can be produced, and that the consonances, which the composers call perfect, are fashioned after the harmonic division of this number. Because when the consonances are transferred to a sounding body with the aid of the consonant ratios 60:30:20:15:12:10, then one recognizes that these consonances are so divided as the parts of the number 6, although they are now arranged in another manner. Likewise, it is comprehensible in which sense the words of the very learned Jacobus Faber Stapulensis in his “Musica” (Prop. III, 34) are to be understood: that the harmonic proportionality is completely indispensable and that, although the magnitudes of its proportions agree with those of the arithmetic proportionality, the sequence and the place [position] of the ratios are different.[28] (Bold italics, and text and ratios in brackets mine. Italics in Zarlino’s original text. Text in parentheses in Fend’s German translation.)
In the first paragraph of Quote V, Zarlino identifies the “nature of Harmony” — or the very essence of musical harmony — with the harmonic division of strings. His preference for the harmonic division is based on the performance of choral music, where one places large intervals in the bass, or in the lower position of a chord, and small intervals in the treble, or in the upper position of a chord. In the second paragraph, Zarlino establishes an irrefutable nexus between his numero Senario and Stifel’s harmonic division of length ratio 6/1, notated here as the harmonic progression 60:30:20:15:12:10, or an ascending sequence of interval ratios: 60/30, 30/20, 20/15, 15/12, 12/10, or simply 2/1, 3/2, 4/3, 5/4, 6/5. To strengthen his argument, in the next sentence Zarlino paraphrases a passage from a famous mathematical treatise entitled Musica libris quatuor demonstrata, by Jacobus Faber Stapulensis (Jacques Le Febvre), (c. 1455 – d. 1536), first published in 1496.
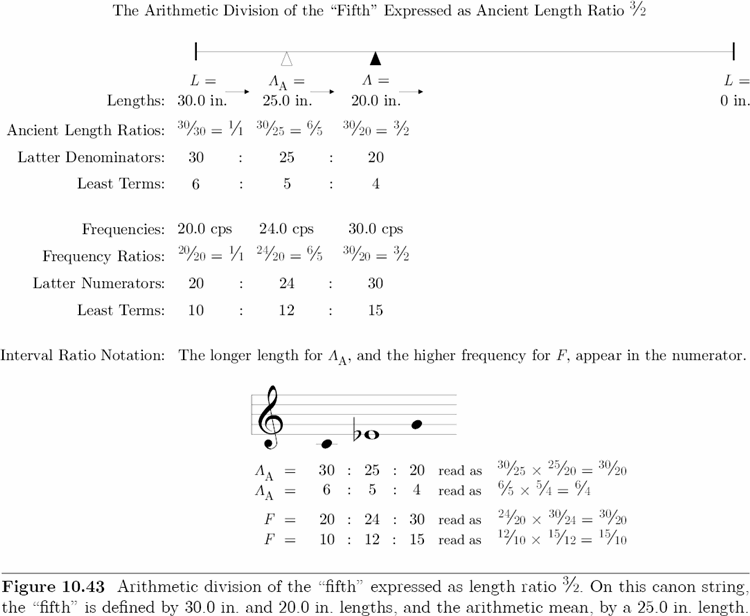
Zarlino’s unrelenting determination to shift the focus from the arithmetic division to the harmonic division reveals how deeply entrenched the practice of direct canon string division had become. As discussed in Sections 10.4 and 10.8, the latter method always produces an arithmetic division, where the smaller interval appears in the lower position, and the larger interval, in the upper position. With respect to the “octave,” it matters little if in playing a chord one utilizes the arithmetic or the harmonic division. The former places the “fourth,” ratio 4/3, in the lower position, and the “fifth,” ratio 3/2, in the upper position; and the latter places the “fifth” in the lower position, and the “fourth” in the upper position. However, with respect to the “fifth,” and what would later be called triadic harmony, the difference between the arithmetic division of length ratio 3/2, and the harmonic division of length ratio 3/2, literally defines the emotional polarity of Western music. Figure 10.43 shows that the arithmetic division of the “fifth” places the “minor third,” ratio 6/5, in the lower position, and the “major third,” ratio 5/4, in the upper position; we call the chord C–Eb–G a minor triad, or minor tonality.
In contrast, Figure 10.44 shows that the harmonic division of the “fifth” places the “major third” in the lower position, and the “minor third” in the upper position; we call the chord C–E–G a major triad, or major tonality.

Now, turn back to Figure 10.39, and note that the minor triad F–Ab–C occurs in Stifel’s arithmetic progression when realized on a string with 6, 5, 4, aliquot parts; and, in Figure 10.40, the major triad C–E–G occurs in Stifel’s harmonic progression when realized on a string with 15, 12, 10, aliquot parts.
Section 10.45
Before we examine Zarlino’s final analysis of the arithmetic and harmonic division of the “fifth,” let us first evaluate two important mathematical aspects of the Senario. In his paper, Robert W. Wienpahl states that “. . . the major and minor sixth are not considered by Zarlino to be basic consonances . . .”[29] because they are not superparticular or epimore ratios. In Section 10.4, we noted that a superparticular ratio has a numerator that exceeds the denominator by one. Therefore, since the integers of the “major sixth,” ratio 5/3, and the “minor sixth,” ratio 8/5, do not differ by unity, Zarlino concludes that they are Composite or imperfect consonances. Zarlino distinguishes between Simple and Composite consonances in the Istitutioni harmoniche, Prima Parte, Cap. 16. Near the end of this chapter, he concludes
Quote VI
In the Senario, that is, in its parts, one finds every Simple musical consonance in atto [lit. in actuality], and beyond that, the Compound [musical consonance] in potenza [lit. in potentiality].[30] (Text in brackets mine. Italics in Zarlino’s original text.)
In the middle of this chapter, Zarlino contends
To the . . . [compound consonances] belongs the mentioned [major] sixth, which consists of the fourth and the major third. It is recognized by the simplest terms of its proportion, 5 and 3, which is divided by 4 into 5:4:3.[31] (Text in brackets mine.)
In other words, because the “major sixth,” expressed as interval ratios 5:4:3, consists of two smaller consonances, Zarlino considers it a composite consonance: 5/4 × 4/3 = 5/3. He then continues by applying the same argument to the “minor sixth”:
Quote VII
Next to it, I will place the minor sixth, which arises from a union of the fourth and the minor third. Its simplest terms are contained in the genus superpartiens as the ratio supertripartiensquinta, and they can be joined by a middle term. Since one finds this proportion between 8 and 5, a middle harmonic number is included between them, namely, the 6. It divides the proportion 8:5 into two smaller ratios 8:6:5, that is, a sesquitertia and a sesquiquinta. For this reason we can characterize this consonance as a Compound [consonance]. Until now, it has received a friendly reception by musicians, and it is counted among the other consonances. If, under the parts of the Senario, one does not come across its form in atto [in actuality], one finds it there nevertheless in potenza [in potentiality]. Because, it builds its form in truth from the parts that are contained in the number 6, that is, from the fourth and the minor third.[32] (Text in brackets mine. Italics in Zarlino’s original text.)
That is, because the “minor sixth,” expressed as interval ratios 8:6:5, also consists of two smaller consonances, it too is a composite consonance: 8/6 × 6/5 = 8/5. Therefore, both the “major sixth” and “minor sixth” are considered less than perfect consonances.
Although the “major sixth” and the “minor sixth” are both superpartient ratios, note that the Senario includes both integers of ratio 5:3, but only one integer of ratio 8:5. Because of this, Zarlino’s rationalization with respect to ratio 5/3 seems unnecessary and contradictory. To resolve this confusion, we may conjecture that if the Senario had contained both integers of ratio 8/5, Zarlino would probably not have given an inconsistent description of ratio 5/3. In other words, the necessity to rationalize the inclusion of superpartient ratio 8/5 forced him to rationalize superpartient ratio 5/3 as well. The inscription in the inner circle of Figure 10.36 is evidence enough that Zarlino primarily regarded ratio 5/3 as a Simple or basic consonance.
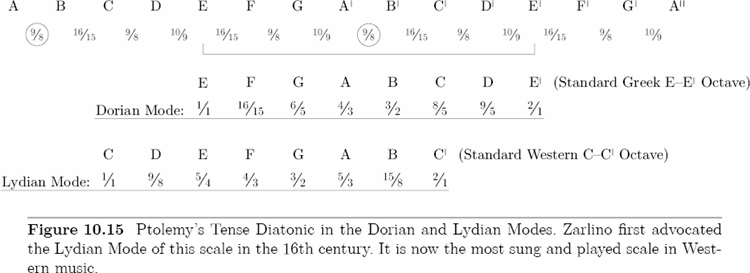
As if to acknowledge his inconsistent treatment of ratio 5/3, Zarlino draws a hard distinction between 5/3 and 8/5 by directly stating in Quote VI, and by indirectly stating in Quote VII, that the former is found “in actuality” in the Senario, but the latter is only found “in potentiality.” When viewed from this perspective, ratio 5/3 is an actual consonance among all the other consonances in Figure 10.36, but ratio 8/5 is only a potential consonance that stands apart from the ratios in this figure. To understand Zarlino’s apparent reluctance to classify ratio 8/5 as a bona fide dissonance, consider this sequence of ratios: 6/5, 4/3, 3/2, 8/5. Now turn back to Section 10.20, and observe that in the Catalog of Scales of the Harmonics by Claudius Ptolemy (c. A.D. 100 – c. 165), only Ptolemy’s Tense Diatonic includes these four ratios. In Section 10.21, Figure 10.15 shows that in the Lydian Mode, the latter sequence transforms to ratios: 5/4, 4/3, 3/2, 5/3.[33] Given Zarlino’s fascination with Ptolemy’s scale, his artistic predilections led him to regard ratios 5/3 and 8/5 as unequal but musically acceptable consonances.
Section 10.46
Toward the end of his life, Zarlino abandoned the needlessly conflicted rhetoric of his early writings. In the De tutte l’opere… edition of the Istitutioni harmoniche he gives equal consideration to the harmonic and the arithmetic division of the “fifth.” With the exception of the modern G-clef, Figure 10.45(a) is an exact copy of Zarlino’s illustration as it appears in De tutte l’opere…, Terza Parte, Cap. 31, p. 222. Figure 10.45(b) gives a detailed ratio analysis of Figure 10.45(a). For the harmonic division of the “pure fifth,” expressed as length ratio 180/120 = 3/2, Zarlino describes the lower interval, ratio 180/144 = 5/4 [386.3 ¢], as a ditono (lit. two-tones) and sesquiquarta; and he describes the upper interval, ratio 144/120 = 6/5 [315.6 ¢], as a semiditono (lit. flat two-tones) and sesquiquinta. To verify his harmonic mean calculation, substitute the outer two terms — 180 units and 120 units — into Equation 3.38a to obtain the units:
![]()
Unfortunately, the arithmetic division of the “fifth” is not so simple. In Figure 10.45(b), observe that the length ratios above the staff represent the first six tones of Ptolemy’s Tense Diatonic in the ancient Lydian Mode: 1/1, 9/8, 5/4, 4/3, 3/2, 5/3; hence, string lengths 180 ÷ 9/8 = 160, 180 ÷ 5/4 = 144, . . . , etc. Given this sequence of tones, the interval between the “whole tone,” ratio 9/8, and the “major sixth,” ratio 5/3, is a “flat fifth”: 5/3 ÷ 9/8 = 40/27 [680.4 ¢], or a “fifth” tuned 1 syntonic comma flat: 3/2 ÷ 81/80 = 40/27. A substitution of the outer two terms — 160 units and 108 units — into Equation 3.37a gives the following arithmetic mean:
![]()
Now, if Zarlino had given this exact result in Figure 10.45(a), it would have produced two very complex interval ratios with prime number 67; that is, a lower “flat minor third,” ratio 160/134 = 80/67 [307.0 ¢], and an upper “flat major third,” ratio 134/108 = 67/54 [373.4 ¢]. He avoids this difficulty by increasing 134 units to 135 units, but also incurs a small mathematical error. The lower interval is now a Pythagorean “minor third,” ratio 160/135 = 32/27 [294.1 ¢] — or a 5-limit “minor third” tuned 1 syntonic comma flat: 6/5 ÷ 81/80 = 32/27 — and the upper interval, the desired 5-limit “major third,” ratio 135/108 = 5/4, or a true sesquiquarta. However, in the final analysis, the “flat fifth” and the Pythagorean “minor third” approximations do not contradict Zarlino’s original intent, namely, to demonstrate the major and minor tonalities in the context of a single musical scale.
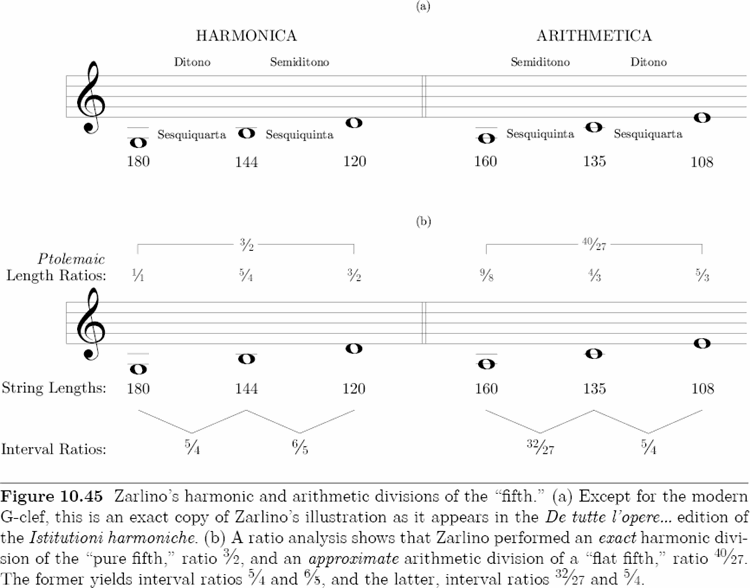
To clarify the musical distinction between the harmonic division of the “fifth” and major tonality on the one hand, and the arithmetic division of the “fifth” and minor tonality on the other, Zarlino describes Figure 10.45(a) by stating
Quote VIII
. . . the variety of the harmony . . . consists not only in the variety of the consonances which occur between the parts, but also in the variety of the harmonies, which arises from the position of the sound forming the third or tenth above the lowest part of the composition. Either this is minor and the resulting harmony is ordered by or resembles the arithmetical proportion or [arithmetic] mean, or it is major and the harmony is ordered by or resembles the harmonic [proportion].
On this variety depend the whole diversity and perfection of the harmonies. For . . . in the perfect composition the fifth and third, or their extensions [or “octave” equivalents; i.e., the “twelfth” and “tenth,” respectively], must always be actively present, seeing that apart from these two consonances the ear can desire no sound that falls between their extremes or beyond them and yet is wholly distinct and different from those that lie within the extremes of these two consonances combined. For in this combination occur all the different sounds that can form different harmonies.[34] (Italics, bold italics, and text in brackets mine.)
He then continues by attributing a “joyful” sensibility to the major tonality, and a “mournful” sensibility to the minor tonality.
Quote IX
But since the extremes of the fifth are invariable and always placed subject to the same proportion, apart from certain cases in which the fifth is used imperfectly, the extremes of the thirds are given different positions. I do not say different in proportion; I say different in position, for . . . when the major third is placed below, the harmony is made joyful, and when it is placed above, the harmony is made mournful. Thus, from the different positions of the thirds which are placed in counterpoint between the extremes of the fifth or above the octave, the variety of harmony arises.[35] (Bold italics mine.)
We conclude that the Senario not only enabled Zarlino to define a theory of consonance, but also provided him with two mathematical means to describe the polar emotions of human existence.
Stifel, who also recognized the musical importance of both means, was not swayed by the rhetorical arguments of his day. He wrote in the Arithmetica integra:
But I do not see what the Harmonic [progression] may explain about musical concords that the Arithmetic [progression] does not explain in equal proportion [i.e., just as well].[36] (Text in brackets mine.)
Zarlino’s contributions to Western music are truly monumental. By integrating the mathematical principles of Ptolemy’s scale, Ramis’ monochord, Stifel’s arithmetic and harmonic divisions of length ratio 6/1, and his theory of consonance as defined by the Senario, Zarlino gave Western music its modern roots. Although Zarlino favored 1/4-comma meantone temperament for the tuning of keyboard instruments,[37] his theory of consonance was exclusively based on rational or just intoned ratios. Irrational or tempered ratios do not play any part in the formulation of his musical ideas. Four hundred years later, Western music theory still agrees with the basic premise of the Senario, and teaches that only these ratios constitute desirable consonances.
[1]De tutte l’opere del R.M. Gioseffo Zarlino da Chioggia… is available in a facsimile edition from Georg Olms Verlag, Hildesheim, Germany.
[2]Ibn Sina (Avicenna): Auicene perhypatetici philosophi: ac medicorum facile primi opera in luce redacta… This Latin translation was published in 1508. Facsimile Edition: Minerva, Frankfurt am Main, Germany, 1961.
The second chapter (or book) of this compendium is entitled Sufficientia.
[3]Facsimile editions or translations of Arithmetica integra are not available. See Sections 10.43 and 10.46, and Chapter 9, Footnote 6, for translated excerpts from this work. Stifel was the first mathematician to use the term exponent, and to state the four laws of exponents.
[4](a) Zarlino, R.M.G. (1571). Dimostrationi harmoniche, Ragionamento Terzo, Proposta VIIII (i.e., IX), pp. 158–160. Facsimile Edition, The Gregg Press Incorporated, Ridgewood, New Jersey, 1966.
(b) Kelleher, J.E. (1993). Zarlino’s “Dimostrationi harmoniche” and Demonstrative Methodologies in the Sixteenth Century, pp. 265–268. Ph.D. dissertation printed and distributed by University Microfilms, Inc., Ann Arbor, Michigan.
This dissertation includes many translated excerpts from Zarlino’s Dimostrationi. Here Kelleher describes not only Zarlino’s criticism, but also quotes several translated passages from Stifel’s Arithmetica integra.
[5]See Chapter 3, Section 12.
[6]D’Erlanger, R., Bakkouch, ‘A.‘A., and Al-Sanusi, M., Translators (Vol. 1, 1930; Vol. 2, 1935; Vol. 3, 1938; Vol. 4, 1939; Vol. 5, 1949; Vol. 6, 1959). La Musique Arabe, Librairie Orientaliste Paul Geuthner, Paris, France.
Forster Translation: in La Musique Arabe, Volume 1, pp. 100–101.
[7]See Chapter 11, Part IV.
[8]Reichenbach, H. (1951). The Rise of Scientific Philosophy. The University of California Press, Berkeley and Los Angeles, California, 1958.
[9](a) Fend, M., Translator (1989). Theorie des Tonsystems: Das erste und zweite Buch der Istitutioni harmoniche (1573), von Gioseffo Zarlino. Peter Lang, Frankfurt am Main, Germany.
This German translation includes the Prima and Seconda Parte of the Istitutioni harmoniche.
Forster Translation: in Theorie des Tonsystems, pp. 104–105.
(b) Zarlino R.M.G. (1573). Istitutioni harmoniche. Facsimile Edition, The Gregg Press Limited, Farnborough, Hants., England, 1966.
Forster Translation: in Istitutioni harmoniche, pp. 37–38.
[10]Auicene perhypatetici philosophi . . . I, Cap. 8, p. 18.
[11]Farmer, H.G. (1965). The Sources of Arabian Music, p. 36. E.J. Brill, Leiden, Netherlands.
[12]Forster Translation: in La Musique Arabe, Volume 2, p. 124.
With respect to the “puissance” translation error, see Al-Farabi’s ‘Uds, Footnote 22.
[13]Levin, F.R., Translator (1994). The Manual of Harmonics, of Nicomachus the Pythagorean, pp. 107–108. Phanes Press, Grand Rapids, Michigan.
[14]Lawlor, R. and D., Translators (1978). Mathematics Useful for Understanding Plato, by Theon of Smyrna, pp. 76–79. Wizards Bookshelf, San Diego, California, 1979.
[15]Bower, C.M., Translator, (1989). Fundamentals of Music, by A.M.S. Boethius, pp. 65–72. Yale University Press, New Haven, Connecticut.
[16]Forster Translation: in La Musique Arabe, Volume 2, p. 136.
[17]Forster Translation: in La Musique Arabe, Volume 2, pp. 136–137.
[18]Forster Translation: in La Musique Arabe, Volume 3, pp. 36–37.
[19]Forster Translation: in Theorie des Tonsystems, p. 87; in Istitutioni harmoniche, p. 31.
[20]Forster Translation: in Theorie des Tonsystems, pp. 87–88; in Istitutioni harmoniche, pp. 31–32.
[21]Forster Translation: in Theorie des Tonsystems, pp. 148–149; in Istitutioni harmoniche, pp. 54–55.
[22]Forster Translation: in Theorie des Tonsystems, p. 156; in Istitutioni harmoniche, p. 60.
[23]Forster Translation: in Theorie des Tonsystems, p. 167.
[24]Forster Translation: in Theorie des Tonsystems, p. 161; in Istitutioni harmoniche, p. 61.
[25]Forster Translation: in Theorie des Tonsystems, pp. 161–162; in Istitutioni harmoniche, pp. 61–62.
[26]Forster Translation: in Theorie des Tonsystems, p. 162; in Istitutioni harmoniche, p. 62.
[27]Forster Translation: in Theorie des Tonsystems, pp. 162–163; in Istitutioni harmoniche, p. 62.
[28]Forster Translation: in Theorie des Tonsystems, pp. 163–164; in Istitutioni harmoniche, pp. 62–63.
[29]Wienpahl, R.W. (1959). Zarlino, the Senario, and tonality, p. 31. Journal of the American Musicological Society XII, No. 1, pp. 27–41.
Wienpahl gives a detailed analysis of the “major sixth” and “minor sixth” in Zarlino’s Senario. This paper also includes many translated excerpts from Zarlino’s Istitutioni harmoniche, and from Salinas’ De musica libri VII.
[30]Forster Translation: in Theorie des Tonsystems, p. 93; in Istitutioni harmoniche, p. 34.
[31]Forster Translation: in Theorie des Tonsystems, p. 91; in Istitutioni harmoniche, p. 33.
[32]Forster Translation: in Theorie des Tonsystems, pp. 91–92; in Istitutioni harmoniche, pp. 33–34.
[33]Zarlino recognized the musical importance of Ptolemy’s Tense Diatonic in the Lydian Mode and passionately advocated its implementation. In the De tutte l’opere… edition of the Istitutioni harmoniche, Seconda Parte, Cap. 39, Zarlino described Ptolemy’s Tense Diatonic Tetrachord as the most natural of all diatonic scales. Today we know it as the just-intoned version of the Western major scale.
[34](a) Strunk, O., Editor (1950). Source Readings in Music History, p. 242. W. W. Norton & Company, Inc., New York.
(b) Journal of the American Musicological Society XII, No. 1, p. 27.
(c) Shirlaw, M. (1917). The Theory of Harmony, p. 50. Da Capo Press Reprint Edition. Da Capo Press, New York, 1969.
[35](a) Source Readings in Music History, pp. 242–243.
(b) Journal of the American Musicological Society XII, No. 1, p. 28.
[36]Forster Translation: in Theorie des Tonsystems, p. 168.
In his commentaries, Fend gives Stifel’s original Latin text: “Non enim video quod Harmonica habeat quod ad concentus Musicos pertineat, quod Arithmetica non habeat aequali commoditate.”
[37]See Section 10.27.